Advertisements
Advertisements
प्रश्न
The electric field in a region is given by `vec"E"` = 5 `hatk`N/C. Calculate the electric flux Through a square of side 10.0 cm in the following cases
- The square is along the XY plane
- The square is along XZ plane
- The normal to the square makes an angle of 45° with the Z axis.
Advertisements
उत्तर
Given: `vec"E"` = 5 `hatk` N/C, |E| = 5 N/C, l = 10 cm = 10 × 10−2 m = 10−1 m, A = l2 = 10−2m2
To find: Electric flux in three cases (Φ1), (Φ2), (Φ3)
(i) Given the plane of Area is along XY plane. hence the direction of its normal `(vec"dS")` vector will be along Z axis.
The Angle between `vec"E"` and `"d"vec"S"` is zero i.e. θ = 0°.
∴ Φ = EA cos θ
∴ Φ1 = 5 × 10−2 cos 0
∴ Φ1 = 5 × 10−2 V m
(ii) Plane of Area is along XZ plane. Hence the direction of its normal `(vec"dS")` vector will be along Y axis.
∴ θ = 90°
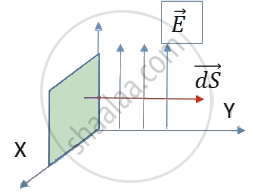
∴ Φ = EA cos θ
∴ Φ2 = 5 × 10−2 cos 90°
∴ Φ2 = 0 V m
(iii) When normal to the square makes an angle of 45° with the Z axis.
∴ θ = 45°
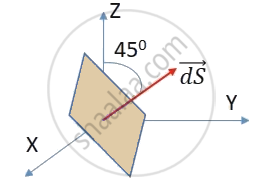
∴ Φ = EA cos θ
∴ Φ3 = 5 × 10−2 cos 45°
∴ Φ3 = 3.5 × 10−2 V m
Electric flux in the above-mentioned cases are 5 × 10−2 V m, 0 V m, and 3.5 × 10−2 V m.
APPEARS IN
संबंधित प्रश्न
Define electric flux.
Find out the outward flux to a point charge +q placed at the centre of a cube of side ‘a’. Why is it found to be independent of the size and shape of the surface enclosing it? Explain.
What is the electric flux through a cube of side 1 cm which encloses an electric dipole?
Given a uniform electric field `vecE=5xx10^3hati`N/C, find the flux of this field through a square of 10 cm on a side whose plane is parallel to the y-z plane. What would be the flux through the same square if the plane makes a 30° angle with the x-axis ?
Consider two hollow concentric spheres, S1 and S2, enclosing charges 2Q and 4Q respectively as shown in the figure. (i) Find out the ratio of the electric flux through them. (ii) How will the electric flux through the sphere S1 change if a medium of dielectric constant 'εr' is introduced in the space inside S1 in place of air ? Deduce the necessary expression
Careful measurement of the electric field at the surface of a black box indicates that the net outward flux through the surface of the box is 8.0 × 103 N m2/C.
- What is the net charge inside the box?
- If the net outward flux through the surface of the box were zero, could you conclude that there were no charges inside the box? Why or Why not?
A uniformly charged conducting sphere of 2.4 m diameter has a surface charge density of 80.0 μC/m2.
- Find the charge on the sphere.
- What is the total electric flux leaving the surface of the sphere?
Given a uniform electric field \[\vec{E} = 2 \times {10}^3 \ \hat{i}\] N/C, find the flux of this field through a square of side 20 cm, whose plane is parallel to the y−z plane. What would be the flux through the same square, if the plane makes an angle of 30° with the x−axis ?
Given a uniform electric filed \[\vec{E} = 4 \times {10}^3 \ \hat{i} N/C\]. Find the flux of this field through a square of 5 cm on a side whose plane is parallel to the Y-Z plane. What would be the flux through the same square if the plane makes a 30° angle with the x-axis?
Two charges of magnitudes −2Q and +Q are located at points (a, 0) and (4a, 0) respectively. What is the electric flux due to these charges through a sphere of radius ‘3a’ with its centre at the origin?
Two charges of magnitudes −3Q and + 2Q are located at points (a, 0) and (4a, 0) respectively. What is the electric flux due to these charges through a sphere of radius ‘5a’ with its centre at the origin?
Two charges of magnitudes +4Q and − Q are located at points (a, 0) and (− 3a, 0) respectively. What is the electric flux due to these charges through a sphere of radius ‘2a’ with its centre at the origin?
A small plane area is rotated in an electric field. In which orientation of the area, is the flux of the electric field through the area maximum? In which orientation is it zero?
A circular ring of radius r made of a non-conducting material is placed with its axis parallel to a uniform electric field. The ring is rotated about a diameter through 180°. Does the flux of the electric field change? If yes, does it decrease or increase?
Following Figure (a) shows an imaginary cube of edge L/2. A uniformly charged rod of length (L) moves towards the left at a small but constant speed `nu.` At t = 0, the left end just touches the centre of the face of the cube opposite it. Which of the graphs shown in the figure (b) represents the flux of the electric field through the cube as the rod goes through it?
The following figure shows a closed surface that intersects a conducting sphere. If a positive charge is placed at point P, the flux of the electric field through the closed surface
An electric charge q is placed at the centre of a cube of side a. The electric flux on one of its faces will be ______.
Total electric flux coming out of a unit positive charge kept in air is ______.
The electric field intensity due to an infinite cylinder of radius R and having charge q per unit length at a distance rir r(r > R) from its axis is ______.
A point charge q is placed at a distance a/2 directly above the centre of a square of side a. The electric flux through the square is ______.
The S.I. unit of electric flux is ______
The electric charges are distributed in a small volume. The flux of the electric field through a spherical surface of radius 10 cm surrounding the total charge is 20 V-m. The flux over a concentric sphere of radius 20 cm will be ______.
A hollow cylinder has a charge of 'q' C within it. If 𝜙 is the electric flux associated with the curved surface B, the flux linked with the plane surface A will be ______.
An electric field is given by `(6 hat i + 5 hat j + 3 hat k)` N/C. The electric flux through a surface area `30 hat i` m2 lying in YZ-plane (in SI unit) is ______.
