Advertisements
Advertisements
प्रश्न
A chord of length 14 cm is at a distance of 6 cm from the centre of a circle. The length of another chord at a distance of 2 cm from the centre of the circle is
पर्याय
12 cm
12 cm
12 cm
18 cm
Advertisements
उत्तर
18 cm
We are given the chord of length 14 cm and perpendicular distance from the centre to the chord is 6 cm. We are asked to find the length of another chord at a distance of 2 cm from the centre.
We have the following figure
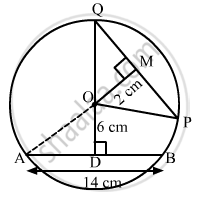
We are given AB = 14 cm, OD = 6 cm, MO = 2 cm, PQ = ?
Since, perpendicular from centre to the chord divide the chord into two equal parts
Therefore
`AQ^2 = AD^2 +OD^2`
= ` 7^2 + 6^2`
= 49 + 36
`= sqrt( 85)`
Now consider the ΔOPQ in which OM = 2 cm
So using Pythagoras Theorem in ΔOPM
`PM^2 = OP^2 -OM^2`
`=(sqrt(85))^2 - 2^2` (∵ OP = AO = radius)
= 81
= 9 cm
Hence PQ = 2 PM
= 2 × 9
= 18 cm
APPEARS IN
संबंधित प्रश्न
Fill in the blanks:
A point, whose distance from the centre of a circle is greater than its radius lies in __________ of the circle. (exterior/ interior)
Prove that the intercept of a tangent between two parallel tangents to a circle subtends a right angle at center.
In the given figure, ABCD is a cyclic quadrilateral. If ∠BCD = 100° and ∠ABD = 70°, find ∠ADB.
In the given figure, BC is a tangent to the circle with centre O. OE bisects AP. Prove that ΔAEO ∼ Δ ABC.
In the given figure, PA and PB are tangents to the circle from an external point P. CD is another tangent touching the circle at Q. If PA = 12 cm, QC = QD = 3 cm, then find PC + PD.
Draw a circle of radius 6 cm. In the circle, draw a chord AB = 6 cm.
(i) If O is the center of the circle, join OA and OB.
(ii) Assign a special name to ∆AOB
(iii) Write the measure of angle AOB.
Find the length of the chord AC where AB and CD are the two diameters perpendicular to each other of a circle with radius `4sqrt(2)` cm and also find ∠OAC and ∠OCA
Circles with centers A, B and C touch each other externally. If AB = 36, BC = 32, CA = 30, then find the radii of each circle.
A point P is 10 cm from the center of a circle. The length of the tangent drawn from P to the circle is 8 cm. The radius of the circle is equal to ______
If two chords AB and CD of a circle AYDZBWCX intersect at right angles (see figure), prove that arc CXA + arc DZB = arc AYD + arc BWC = semi-circle.
