Advertisements
Advertisements
प्रश्न
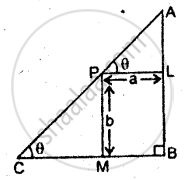
A point on the hypotenuse of a triangle is at distance a and b from the sides of the triangle.
Show that the minimum length of the hypotenuse is `(a^(2/3) + b^(2/3))^(3/2).`
उत्तर
Let P be a point on the hypotenuse of ∆ABC.
Draw perpendicular PL from P on AB and PM from P on BC.
Let ∠ACB = θ = ∠APL
AP = a sec θ, PC = b cosec θ
Let the length of the hypotenuse be l, then
l = AP + PC
= a sec θ + b cosec θ
On differentiating with respect to θ,
`therefore (dl)/(d theta) = a sec theta tan theta - b cosec theta cot theta`
For maximum and minimum, `(dl)/(d theta) = 0`
⇒ a sec θ tan θ - b cosec θ cot θ = 0
`=> a 1/(cos θ) * (sin θ)/(cos θ) - b 1/(sin θ) * (cos θ)/(sin θ) = 0`
`=> (a sin θ)/(cos^2 θ) - (b cos θ)/(sin^2 θ) = 0`
⇒ a sin3 θ - b cos3 θ = 0
⇒ a sin3 θ = b cos3 θ
`=> (sin^3 θ)/(cos^3 θ) = b/a`
`=> tan^3 θ = b/a`
`=> tan θ = (b/a)^(1//3)`
On differentiating again,
`(d^2l)/(d theta) = a (sec θ * sec^2 θ + tan θ * sec θ tan θ) - b [cosec θ (- cosec^2 θ) + cot θ (- cosec θ cot θ)]`
`= a sec θ (sec^2 θ + tan^2 θ) + b cosec θ xx (cosec^2 θ xx cot^2 θ)`
`because 0 < theta < pi/2` So all trigonometric ratios of θ are positive.

`therefore (d^2 l)/(d theta)^2` = + ve i.e. l is minimum.
a > 0 and b > 0
`therefore (d^2 l)/(d theta)^2` = + ve
When `tan theta = (b/a)^(1/3)` then l is minimum.
∴ Minimum value of l = a sec θ + b cosec θ
`= a sqrt (a^(2/3) + b^(2/3))/(a^(1/3)) + b sqrt (a^(2/3) + b^(2/3))/ b^(1/3)`
`= sqrt (a^(2/3) + b^(2/3)) (a^(2/3) + b^(2/3))`
`= (a^(2/3) + b^(2/3))^(3/2).`
APPEARS IN
संबंधित प्रश्न
Find the maximum and minimum value, if any, of the following function given by f(x) = (2x − 1)2 + 3.
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
`h(x) = sinx + cosx, 0 < x < pi/2`
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
f(x) = x3 − 6x2 + 9x + 15
Find the absolute maximum value and the absolute minimum value of the following function in the given interval:
`f(x) =x^3, x in [-2,2]`
At what points in the interval [0, 2π], does the function sin 2x attain its maximum value?
Find the maximum value of 2x3 − 24x + 107 in the interval [1, 3]. Find the maximum value of the same function in [−3, −1].
Find the maximum and minimum values of x + sin 2x on [0, 2π].
The point on the curve x2 = 2y which is nearest to the point (0, 5) is ______.
Show that a cylinder of a given volume, which is open at the top, has minimum total surface area when its height is equal to the radius of its base.
Find the maximum and minimum of the following functions : f(x) = `logx/x`
A ball is thrown in the air. Its height at any time t is given by h = 3 + 14t – 5t2. Find the maximum height it can reach.
The profit function P(x) of a firm, selling x items per day is given by P(x) = (150 – x)x – 1625 . Find the number of items the firm should manufacture to get maximum profit. Find the maximum profit.
Determine the maximum and minimum value of the following function.
f(x) = 2x3 – 21x2 + 36x – 20
Determine the maximum and minimum value of the following function.
f(x) = x log x
Max value of z equals 3x + 2y subject to x + y ≤ 3, x ≤ 2, -2x + y ≤ 1, x ≥ 0, y ≥ 0 is ______
Twenty meters of wire is available for fencing off a flowerbed in the form of a circular sector. Then the maximum area (in sq. m) of the flower-bed, is ______
The sum of two non-zero numbers is 6. The minimum value of the sum of their reciprocals is ______.
Show that the function f(x) = 4x3 – 18x2 + 27x – 7 has neither maxima nor minima.
Find the points of local maxima, local minima and the points of inflection of the function f(x) = x5 – 5x4 + 5x3 – 1. Also find the corresponding local maximum and local minimum values.
The maximum value of `["x"("x" − 1) + 1]^(1/3)`, 0 ≤ x ≤ 1 is:
If y = x3 + x2 + x + 1, then y ____________.
Find the height of the cylinder of maximum volume that can be inscribed in a sphere of radius a.
The range of a ∈ R for which the function f(x) = `(4a - 3)(x + log_e5) + 2(a - 7)cot(x/2)sin^2(x/2), x ≠ 2nπ, n∈N` has critical points, is ______.
A wire of length 36 m is cut into two pieces, one of the pieces is bent to form a square and the other is bent to form a circle. If the sum of the areas of the two figures is minimum, and the circumference of the circle is k (meter), then `(4/π + 1)`k is equal to ______.
If p(x) be a polynomial of degree three that has a local maximum value 8 at x = 1 and a local minimum value 4 at x = 2; then p(0) is equal to ______.
If the function y = `(ax + b)/((x - 4)(x - 1))` has an extremum at P(2, –1), then the values of a and b are ______.
The set of values of p for which the points of extremum of the function f(x) = x3 – 3px2 + 3(p2 – 1)x + 1 lie in the interval (–2, 4), is ______.
The minimum value of 2sinx + 2cosx is ______.
The volume of the greatest cylinder which can be inscribed in a cone of height 30 cm and semi-vertical angle 30° is ______.
Sum of two numbers is 5. If the sum of the cubes of these numbers is least, then find the sum of the squares of these numbers.
Complete the following activity to divide 84 into two parts such that the product of one part and square of the other is maximum.
Solution: Let one part be x. Then the other part is 84 - x
Letf (x) = x2 (84 - x) = 84x2 - x3
∴ f'(x) = `square`
and f''(x) = `square`
For extreme values, f'(x) = 0
∴ x = `square "or" square`
f(x) attains maximum at x = `square`
Hence, the two parts of 84 are 56 and 28.
The rectangle has area of 50 cm2. Complete the following activity to find its dimensions for least perimeter.
Solution: Let x cm and y cm be the length and breadth of a rectangle.
Then its area is xy = 50
∴ `y =50/x`
Perimeter of rectangle `=2(x+y)=2(x+50/x)`
Let f(x) `=2(x+50/x)`
Then f'(x) = `square` and f''(x) = `square`
Now,f'(x) = 0, if x = `square`
But x is not negative.
∴ `x = root(5)(2) "and" f^('')(root(5)(2))=square>0`
∴ by the second derivative test f is minimum at x = `root(5)(2)`
When x = `root(5)(2),y=50/root(5)(2)=root(5)(2)`
∴ `x=root(5)(2) "cm" , y = root(5)(2) "cm"`
Hence, rectangle is a square of side `root(5)(2) "cm"`
A right circular cylinder is to be made so that the sum of the radius and height is 6 metres. Find the maximum volume of the cylinder.
If x + y = 8, then the maximum value of x2y is ______.
Divide the number 100 into two parts so that the sum of their squares is minimum.
Sumit has bought a closed cylindrical dustbin. The radius of the dustbin is ‘r' cm and height is 'h’ cm. It has a volume of 20π cm3.
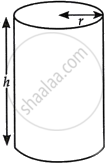
- Express ‘h’ in terms of ‘r’, using the given volume.
- Prove that the total surface area of the dustbin is `2πr^2 + (40π)/r`
- Sumit wants to paint the dustbin. The cost of painting the base and top of the dustbin is ₹ 2 per cm2 and the cost of painting the curved side is ₹ 25 per cm2. Find the total cost in terms of ‘r’, for painting the outer surface of the dustbin including the base and top.
- Calculate the minimum cost for painting the dustbin.
