Question

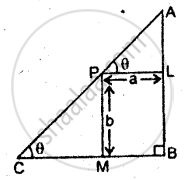
A point on the hypotenuse of a triangle is at distance a and b from the sides of the triangle.

Show that the minimum length of the hypotenuse is `(a^(2/3) + b^(2/3))^(3/2).`

Answer 1

Let P be a point on the hypotenuse of ∆ABC.

Draw perpendicular PL from P on AB and PM from P on BC.

Let ∠ACB = θ = ∠APL

AP = a sec θ, PC = b cosec θ

Let the length of the hypotenuse be l, then

l = AP + PC

= a sec θ + b cosec θ

On differentiating with respect to θ,

`therefore (dl)/(d theta) = a sec theta tan theta - b  cosec  theta cot theta`

For maximum and minimum, `(dl)/(d theta) = 0`

⇒ a sec θ tan θ - b cosec θ cot θ = 0

`=> a 1/(cos θ) * (sin θ)/(cos θ) - b 1/(sin θ) * (cos θ)/(sin θ) = 0`

`=> (a sin θ)/(cos^2 θ) - (b cos θ)/(sin^2 θ) = 0`

⇒ a sin³ θ - b cos³ θ = 0

⇒ a sin³ θ = b  cos³ θ

`=> (sin^3 θ)/(cos^3 θ) = b/a`

`=> tan^3 θ = b/a`

`=> tan θ = (b/a)^(1//3)`

On differentiating again,

`(d^2l)/(d theta) = a (sec θ * sec^2 θ + tan θ * sec θ tan θ) - b [cosec  θ (- cosec^2 θ) + cot θ (- cosec  θ cot θ)]`

`= a sec θ (sec^2 θ + tan^2 θ) + b  cosec  θ xx (cosec^2 θ xx cot^2 θ)`

`because 0 < theta < pi/2` So all trigonometric ratios of θ are positive.

`therefore (d^2 l)/(d theta)^2` = + ve i.e. l ​​is minimum.

a > 0 and b > 0

`therefore (d^2 l)/(d theta)^2` = + ve

When `tan theta = (b/a)^(1/3)` then l is minimum.

∴ Minimum value of l = a sec θ + b cosec θ

`= a sqrt (a^(2/3) + b^(2/3))/(a^(1/3)) + b sqrt (a^(2/3) + b^(2/3))/ b^(1/3)`

`= sqrt (a^(2/3) + b^(2/3)) (a^(2/3) + b^(2/3))`

`= (a^(2/3) + b^(2/3))^(3/2).`