Advertisements
Advertisements
प्रश्न
Answer the following :
Find the length of the tangent segment drawn from the point (5, 3) to the circle x2 + y2 + 10x – 6y – 17 = 0
उत्तर १
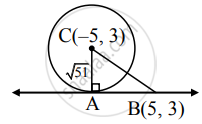
Given equation of circle is
x2 + y2 + 10x – 6y – 17 = 0
Comparing this equation with
x2 + y2 + 2gx + 2fy + c = 0, we get
2g = 10, 2f = –6, c = –17
∴ g = 5, f = –3, c = –17
Centre of circle = (– g, – f )
= C(– 5, 3)
Radius of circle = `sqrt("g"^2 + "f"^2 - "c")`
= `sqrt(5^2 + (-3)^2 - (-17))`
= `sqrt(25 + 9 + 17)`
= `sqrt(51)`
BC = `sqrt((-5 - 5)^2 + (3 - 3)^2`
= `sqrt(100 + 0)`
= 10
In right angled ΔABC
BC2 = AB2 + AC2 …[Pythagoras theorem]
∴ (10)2 = `"AB"^2 + (sqrt(51))^2`
∴ AB2 = 100 – 51 = 49
∴ AB = 7
∴ Length of the tangent segment from (5, 3) is 7 units.
उत्तर २
Given equation of circle is
x2 + y2 + 10x – 6y – 17 = 0
Here, g = 5, f = –3, c = –17
Length of the tangent segment to the circle
x2 + y2 + 2gx + 2fy + c = 0 from the point
(x1, y1) is `sqrt(x_1^2 + y_1^2 + 2"g"x_1 + 2"f"y_1 + "c")`.
∴ Length of the tangent segment from (5, 3)
= `sqrt((5)^2 + (3)^2 + 10(5) - 6(3) - 17)`
= `sqrt(25 + 9 + 50 - 18 - 17)`
= `sqrt(49)`
= 7 units
APPEARS IN
संबंधित प्रश्न
Find the equation of the circle with centre at (−3, −3) passing through the point (−3, −6)
Find the centre and radius of the circle:
(x − 5)2 + (y − 3)2 = 20
Find the equation of the circle with centre at (a, b) touching the Y-axis
Find the equation of the circle with centre at (–2, 3) touching the X-axis.
Find the equation circle if the equations of two diameters are 2x + y = 6 and 3x + 2y = 4. When radius of circle is 9
Find the equation of a circle with radius 4 units and touching both the co-ordinate axes having centre in third quadrant.
Find the equation of a circle passing through the points (1,−4), (5,2) and having its centre on the line x − 2y + 9 = 0
Find the centre and radius of the following:
x2 + y2 − 6x − 8y − 24 = 0
Find the centre and radius of the following:
4x2 + 4y2 − 24x − 8y − 24 = 0
Show that the equation 3x2 + 3y2 + 12x + 18y − 11 = 0 represents a circle
Show that the points (3, −2), (1, 0), (−1, −2) and (1, −4) are concyclic
Choose the correct alternative:
Equation of a circle which passes through (3, 6) and touches the axes is
Choose the correct alternative:
If the lines 2x − 3y = 5 and 3x − 4y = 7 are the diameters of a circle of area 154 sq. units, then find the equation of the circle
Choose the correct alternative:
Area of the circle centre at (1, 2) and passing through (4, 6) is
Choose the correct alternative:
If a circle passes through the point (0, 0), (a, 0) and (0, b) then find the co-ordinates of its centre
Choose the correct alternative:
The equation of a circle with origin as centre and passing through the vertices of an equilateral triangle whose median is of length 3a is
Answer the following :
Find the equation of circle passing through the point of intersection of the lines x + 3y = 0 and 2x − 7y = 0 whose centre is the point of intersection of lines x + y + 1 = 0 and x − 2y + 4 = 0
Answer the following :
Show that the points (9, 1), (7, 9), (−2, 12) and (6, 10) are concyclic
The line 2x − y + 6 = 0 meets the circle x2 + y2 + 10x + 9 = 0 at A and B. Find the equation of circle on AB as diameter.
Answer the following :
Show that the circles touch each other externally. Find their point of contact and the equation of their common tangent:
x2 + y2 – 4x + 10y +20 = 0,
x2 + y2 + 8x – 6y – 24 = 0.
Answer the following :
Show that the circles touch each other externally. Find their point of contact and the equation of their common tangent:
x2 + y2 – 4x – 10y + 19 = 0,
x2 + y2 + 2x + 8y – 23 = 0.
Answer the following :
Show that the circles touch each other internally. Find their point of contact and the equation of their common tangent:
x2 + y2 – 4x – 4y – 28 = 0,
x2 + y2 – 4x – 12 = 0
If one of the diameters of the curve x2 + y2 - 4x - 6y + 9 = 0 is a chord of a circle with centre (1, 1), then the radius of this circle is ______
The centre of the circle x = 3 + 5 cos θ, y = - 4 + 5 sin θ, is ______
The radius of a circle is increasing uniformly at the rate of 2.5cm/sec. The rate of increase in the area when the radius is 12cm, will be ______
The equation of circle whose diameter is the line joining the points (–5, 3) and (13, –3) is ______.
Circle x2 + y2 – 4x = 0 touches ______.
