Advertisements
Advertisements
प्रश्न
Answer the following:
Solve : `sqrt(log_2 x^4) + 4log_4 sqrt(2/x)` = 2
उत्तर
`sqrt(log_2 x^4) + 4log_4 sqrt(2/x)` = 2
∴ `sqrt(4log_2x) + (4log_2(sqrt(2/x)))/log_2 4` = 2
∴ `2sqrt(log_2x) + (4 xx 1/2log_2 (2/x))/(2log_2 2)` = 2
∴ `2sqrt(log_2x) + log_2 (2/x)` = 2 ...[∵ log22 = 1]
∴ `2sqrt(log_2x) + log_2 2 - log_2 x` = 2
∴ `2sqrt(log_2 x) + 1 - log_2 x` = 2
∴ `2sqrt(log_2 x)` = 1 + log2x
Squaring both sides, we get,
4 log2x = (1 + log2x)2
∴ 4 log2x = 1 + 2log2x + (log2x)2
∴ (log2x)2 – 2log2x + 1 = 0
∴ (log2x – 1)2 = 0
∴ log2x – 1 = 0
∴ log2x = 1 = log22
∴ x = 2.
APPEARS IN
संबंधित प्रश्न
A function f : R → R is defined by f(x) = x2. Determine (a) range of f, (b) {x : f(x) = 4}, (c) [y: f(y) = −1].
et A = (12, 13, 14, 15, 16, 17) and f : A → Z be a function given by
f(x) = highest prime factor of x.
Find range of f.
Let A = [p, q, r, s] and B = [1, 2, 3]. Which of the following relations from A to B is not a function?
If \[f\left( x \right) = \frac{x - 1}{x + 1}\] , then show that
(i) \[f\left( \frac{1}{x} \right) = - f\left( x \right)\]
(ii) \[f\left( - \frac{1}{x} \right) = - \frac{1}{f\left( x \right)}\]
If for non-zero x, af(x) + bf \[\left( \frac{1}{x} \right) = \frac{1}{x} - 5\] , where a ≠ b, then find f(x).
Write the range of the function f(x) = cos [x], where \[\frac{- \pi}{2} < x < \frac{\pi}{2}\] .
Let \[f\left( x \right) = \frac{\alpha x}{x + 1}, x \neq - 1\] . Then write the value of α satisfying f(f(x)) = x for all x ≠ −1.
Let A = {1, 2, 3} and B = {2, 3, 4}. Then which of the following is a function from A to B?
If \[f\left( x \right) = \log \left( \frac{1 + x}{1 - x} \right) \text{ and} g\left( x \right) = \frac{3x + x^3}{1 + 3 x^2}\] , then f(g(x)) is equal to
If x ≠ 1 and \[f\left( x \right) = \frac{x + 1}{x - 1}\] is a real function, then f(f(f(2))) is
f is a real valued function given by \[f\left( x \right) = 27 x^3 + \frac{1}{x^3}\] and α, β are roots of \[3x + \frac{1}{x} = 12\] . Then,
The domain of definition of \[f\left( x \right) = \sqrt{\frac{x + 3}{\left( 2 - x \right) \left( x - 5 \right)}}\] is
The domain of definition of the function f(x) = log |x| is
The range of the function f(x) = |x − 1| is
Check if the following relation is function:
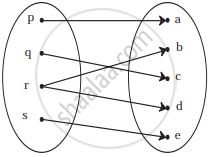
If f(m) = m2 − 3m + 1, find f(0)
Check if the following relation is a function.
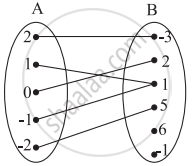
Which sets of ordered pairs represent functions from A = {1, 2, 3, 4} to B = {−1, 0, 1, 2, 3}? Justify.
{(1, 3), (4, 1), (2, 2)}
If f(m) = m2 − 3m + 1, find `f(1/2)`
If f(x) = `("a" - x)/("b" - x)`, f(2) is undefined, and f(3) = 5, find a and b
Find the domain and range of the following function.
g(x) = `(x + 4)/(x - 2)`
Find the domain of f(x) = ln (x − 5)
Solve for x.
log2 x + log4 x + log16 x = `21/4`
Select the correct answer from given alternatives.
If f : R → R is defined by f(x) = x3 then f–1 (8) is equal to :
Select the correct answer from given alternatives.
Let the function f be defined by f(x) = `(2x + 1)/(1 - 3x)` then f–1 (x) is ______.
A function f is defined as : f(x) = 5 – x for 0 ≤ x ≤ 4. Find the value of x such that f(x) = 3
Answer the following:
A function f is defined as : f(x) = 5 – x for 0 ≤ x ≤ 4. Find the value of x such that f(x) = 5
Answer the following:
If f(x) = ax2 + bx + 2 and f(1) = 3, f(4) = 42, find a and b
Answer the following:
Show that, `log |sqrt(x^2 + 1) + x | + log | sqrt(x^2 + 1) - x|` = 0
Given the function f: x → x2 – 5x + 6, evaluate f(2a)
The domain of the real valued function f(x) = `sqrt((x - 2)/(3 - x))` is ______.
If f(x) = `(x - 1)/(x + 1)`, then show that `f(1/x)` = – f(x)
Let f(x) = `sqrt(x)` and g(x) = x be two functions defined in the domain R+ ∪ {0}. Find (f + g)(x)
The domain and range of the real function f defined by f(x) = `(4 - x)/(x - 4)` is given by ______.
If f : R – {2} `rightarrow` R i s a function defined by f(x) = `(x^2 - 4)/(x - 2)`, then its range is ______.
Range of the function f(x) = `x/(1 + x^2)` is ______.
The domain of f(x) = `sin^-1 [log_2(x/2)]` is ______.
