Advertisements
Advertisements
प्रश्न
Select the correct answer from given alternatives.
If f : R → R is defined by f(x) = x3 then f–1 (8) is equal to :
पर्याय
{2}
{–2, 2}
{–2}
(–2, 2)
उत्तर
{2}
Explanation;
f(x) = x3 = y, say
∴ x = `y^(1/3)` = f–1 (y)
∴ f–1 (8) = `(8)^(1/3) = (2^3)^(1/3)`
∴ f–1 (8) = {2}
APPEARS IN
संबंधित प्रश्न
Let f : R+ → R, where R+ is the set of all positive real numbers, such that f(x) = loge x. Determine
(a) the image set of the domain of f
Let X = {1, 2, 3, 4} and Y = {1, 5, 9, 11, 15, 16}
Determine which of the set are functions from X to Y.
(b) f2 = {(1, 1), (2, 7), (3, 5)}
If f(x) = (x − a)2 (x − b)2, find f(a + b).
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(iii) f g
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(v) \[\frac{g}{f}\]
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(viii) \[\frac{5}{8}\]
If f(x) = loge (1 − x) and g(x) = [x], then determine function:
(ii) fg
Let f : [0, ∞) → R and g : R → R be defined by \[f\left( x \right) = \sqrt{x}\] and g(x) = x. Find f + g, f − g, fg and \[\frac{f}{g}\] .
Let f : R → R be defined by f(x) = 2x + |x|. Then f(2x) + f(−x) − f(x) =
If x ≠ 1 and \[f\left( x \right) = \frac{x + 1}{x - 1}\] is a real function, then f(f(f(2))) is
The domain of definition of the function f(x) = log |x| is
If f(m) = m2 − 3m + 1, find f(− x)
A function f is defined as follows: f(x) = 4x + 5, for −4 ≤ x < 0. Find the values of f(−1), f(−2), f(0), if they exist.
Check if the following relation is a function.
Find x, if g(x) = 0 where g(x) = `(5x - 6)/7`
Check the injectivity and surjectivity of the following function.
f : N → N given by f(x) = x3
Check the injectivity and surjectivity of the following function.
f : R → R given by f(x) = x3
Show that if f : A → B and g : B → C are onto, then g ° f is also onto
Express the following exponential equation in logarithmic form
25 = 32
Find the domain of f(x) = ln (x − 5)
Write the following expression as sum or difference of logarithm
In `(("a"^3 ("a" - 2)^2)/sqrt("b"^2 + 5))`
Given that log 2 = a and log 3 = b, write `log sqrt(96)` in terms of a and b
If f(x) = ax2 − bx + 6 and f(2) = 3 and f(4) = 30, find a and b
If `log((x + y)/3) = 1/2 log x + 1/2 logy`, show that `x/y + y/x` = 7
If f(x) = 3x + 5, g(x) = 6x − 1, then find `("f"/"g") (x)` and its domain
Select the correct answer from given alternatives.
If f(x) =`1/(1 - x)`, then f{f[f(x)]} is
Select the correct answer from given alternative.
The domain and range of f(x) = 2 − |x − 5| is
Answer the following:
If f(x) = ax2 + bx + 2 and f(1) = 3, f(4) = 42, find a and b
Answer the following:
Let f : R → R be given by f(x) = x3 + 1 for all x ∈ R. Draw its graph
Answer the following:
If b2 = ac. prove that, log a + log c = 2 log b
Answer the following:
Find the range of the following function.
f(x) = |x – 5|
Answer the following:
Find the range of the following function.
f(x) = `x/(9 + x^2)`
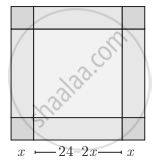
An open box is to be made from a square piece of material, 24 cm on a side, by cutting equal square from the corner and turning up the side as shown. Express the volume V of the box as a function of x
The range of 7, 11, 16, 27, 31, 33, 42, 49 is ______.
If the domain of function f(a) = a2 - 4a + 8 is (-∞, ∞), then the range of function is ______
The domain of the function f(x) = log3+x (x2 - 1) is ______.
Find the domain of the function f given by f(x) = `1/sqrt([x]^2 - [x] - 6)`
Redefine the function f(x) = x − 2 + 2 + x , – 3 ≤ x ≤ 3
Range of f(x) = `1/(1 - 2 cosx)` is ______.
The domain and range of the real function f defined by f(x) = `(4 - x)/(x - 4)` is given by ______.
