Advertisements
Advertisements
प्रश्न
The domain and range of the real function f defined by f(x) =
पर्याय
Domain = R, Range = {–1, 1}
Domain = R – {1}, Range = R
Domain = R – {4}, Range = {– 1}
Domain = R – {– 4}, Range = {–1, 1}
उत्तर
The domain and range of the real function f defined by f(x) =
Explanation:
Given that: f(x) =
We know that f(x) is defined if x – 4 ≠ 0
⇒ x ≠ 4
So, the domain of f(x) is = R – {4}
Let f(x) = y =
⇒ yx – 4y = 4 – x
⇒ yx + x = 4y + 4
⇒ x(y + 1) = 4y + 4
⇒ x =
If x is real number, then 1 + y ≠ 0
⇒ y ≠ – 1
∴ Range of f(x) = R {– 1}
APPEARS IN
संबंधित प्रश्न
Let f : R+ → R, where R+ is the set of all positive real numbers, such that f(x) = loge x. Determine
(a) the image set of the domain of f
If f(x) = x2, find
If f, g, h are real functions given by f(x) = x2, g(x) = tan x and h(x) = loge x, then write the value of (hogof)
Let f and g be two real functions given by
f = {(0, 1), (2, 0), (3, −4), (4, 2), (5, 1)} and g = {(1, 0), (2, 2), (3, −1), (4, 4), (5, 3)}
Find the domain of fg.
If A = {1, 2, 3} and B = {x, y}, then the number of functions that can be defined from A into B is
If
The domain of definition of
The domain of definition of the function f(x) = log |x| is
The range of the function f(x) = |x − 1| is
Which of the following relations are functions? If it is a function determine its domain and range:
{(2, 1), (4, 2), (6, 3), (8, 4), (10, 5), (12, 6), (14, 7)}
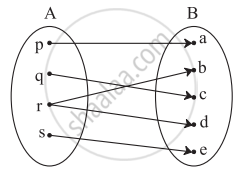
Check if the following relation is a function.
Check if the relation given by the equation represents y as function of x:
3x − 6 = 21
Let f be a subset of Z × Z defined by f = {(ab, a + b) : a, b ∈ Z}. Is f a function from Z to Z? Justify?
Express the following logarithmic equation in exponential form
log10 (0.001) = −3
Express the following logarithmic equation in exponential form
Find the domain of f(x) = log10 (x2 − 5x + 6)
Write the following expression as a single logarithm.
5 log x + 7 log y − log z
Answer the following:
Let f: R → R be a function defined by f(x) = 5x3 – 8 for all x ∈ R, show that f is one-one and onto. Hence find f –1
Answer the following:
If a2 + b2 = 7ab, show that,
Answer the following:
Show that, logy x3 . logz y4 . logx z5 = 60
Answer the following:
Find the domain of the following function.
f(x) =
Answer the following:
Find the domain of the following function.
f(x) =
Answer the following:
Find (f ° g) (x) and (g ° f) (x)
f(x) =
A function f is defined by f(x) = 2x – 3 find x such that f(x) = 0
The data in the adjacent table depicts the length of a person's forehand and their corresponding height. Based on this data, a student finds a relationship between the height (y) and the forehand length (x) as y = ax + b, where a, b are constant.
| Length ‘x’ of forehand (in cm) |
Height 'y' (in inches) |
| 35 | 56 |
| 45 | 65 |
| 50 | 69.5 |
| 55 | 74 |
Check if this relation is a function
If f(x) = 5x - 3, then f-1(x) is ______
Let f and g be two functions given by f = {(2, 4), (5, 6), (8, – 1), (10, – 3)} g = {(2, 5), (7, 1), (8, 4), (10, 13), (11, – 5)} then. Domain of f + g is ______.
Let f(x) =
The ratio
