Advertisements
Advertisements
प्रश्न
Calculate the total torque acting on the body shown in the following figure about the point O.

उत्तर
Torque about a point = Total force × Perpendicular distance
Let the anticlockwise torque and clockwise acting torque be positive and negative, respectively.
Torque at O due to 5 N force is zero as it is passing through O.
Torque at O due to 15 N force,
\[\tau_1 = 15 \times 6 \times {10}^{- 2} \times \sin37^\circ\]
\[ = 15 \times 6 \times {10}^{- 2} \times \frac{3}{5}\]
\[ = 0 . 54 N - m .........\left(\text{anticlockwise} \right)\]
Torque at O due to 10 N force,
\[\tau_2 = 10 \times 4 \times {10}^{- 2} = 0 . 4 N - m ........\left(\text{clockwise} \right)\]
Torque at O due to 20 N force,
\[\tau_3 = 20 \times 4 \times {10}^{- 2} \times \sin30^\circ\]
\[ = 20 \times 4 \times {10}^{- 2} \times \frac{1}{2}\]
\[ = 0 . 4 N - m .........\left(\text{Anticlockwise} \right)\]
Resultant torque acting at O,
\[\tau = \left( 0 . 54 - 0 . 4 + 0 . 4 \right)\]
\[ = 0 . 54 N - m \left(\text{Anticlockwise} \right)\]
APPEARS IN
संबंधित प्रश्न
A solid cylinder of mass 20 kg rotates about its axis with angular speed 100 rad s–1. The radius of the cylinder is 0.25 m. What is the kinetic energy associated with the rotation of the cylinder? What is the magnitude of the angular momentum of the cylinder about its axis?
The torque of the weight of any body about any vertical axis is zero. If it always correct?
A heavy particle of mass m falls freely near the earth's surface. What is the torque acting on this particle about a point 50 cm east to the line of motion? Does this torque produce any angular acceleration in the particle?
If several forces act on a particle, the total torque on the particle may be obtained by first finding the resultant force and then taking torque of this resultant. Prove this. Is this result valid for the forces acting on different particles of a body in such a way that their lines of action intersect at a common point?
If the resultant torque of all the forces acting on a body is zero about a point, is it necessary that it will be zero about any other point?
A body is in translational equilibrium under the action of coplanar forces. If the torque of these forces is zero about a point, is it necessary that it will also be zero about any other point?
When a body is weighed on an ordinary balance we demand that the arum should be horizontal if the weights on the two pans are equal. Suppose equal weights are put on the two pans, the arm is kept at an angle with the horizontal and released. Is the torque of the two weights about the middle point (point of support) zero? Is the total torque zero? If so, why does the arm rotate and finally become horizontal?
A simple pendulum of length l is pulled aside to make an angle θ with the vertical. Find the magnitude of the torque of the weight ω of the bob about the point of suspension. When is the torque zero?
A rope is wound around a hollow cylinder of mass 3 kg and radius 40 cm. What is the angular acceleration of the cylinder if the rope is pulled with a force of 30 N?
The ratio of the acceleration for a solid sphere (mass m and radius R) rolling down an incline of angle θ without slipping and slipping down the incline without rolling is, ______
What are the conditions in which force can not produce torque?
A Merry-go-round, made of a ring-like platform of radius R and mass M, is revolving with angular speed ω. A person of mass M is standing on it. At one instant, the person jumps off the round, radially away from the centre of the round (as seen from the round). The speed of the round afterwards is ______.
Choose the correct alternatives:
- For a general rotational motion, angular momentum L and angular velocity ω need not be parallel.
- For a rotational motion about a fixed axis, angular momentum L and angular velocity ω are always parallel.
- For a general translational motion , momentum p and velocity v are always parallel.
- For a general translational motion, acceleration a and velocity v are always parallel.
A uniform sphere of mass m and radius R is placed on a rough horizontal surface (Figure). The sphere is struck horizontally at a height h from the floor. Match the following:
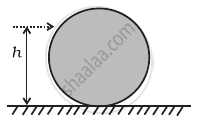
| Column I | Column II | |
| (a) h = R/2 | (i) | Sphere rolls without slipping with a constant velocity and no loss of energy. |
| (b) h = R | (ii) | Sphere spins clockwise, loses energy by friction. |
| (c) h = 3R/2 | (iii) | Sphere spins anti-clockwise, loses energy by friction. |
| (d) h = 7R/5 | (iv) | Sphere has only a translational motion, looses energy by friction. |
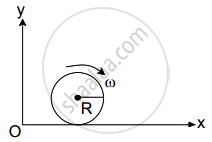
A spherical shell of 1 kg mass and radius R is rolling with angular speed ω on horizontal plane (as shown in figure). The magnitude of angular momentum of the shell about the origin O is `a/3 R^2` ω. The value of a will be:
A particle of mass 'm' is moving in time 't' on a trajectory given by
`vecr = 10alphat^2hati + 5beta(t - 5)hatj`
Where α and β are dimensional constants.
The angular momentum of the particle becomes the same as it was for t = 0 at time t = ______ seconds.
