Advertisements
Advertisements
Question
Calculate the total torque acting on the body shown in the following figure about the point O.

Solution
Torque about a point = Total force × Perpendicular distance
Let the anticlockwise torque and clockwise acting torque be positive and negative, respectively.
Torque at O due to 5 N force is zero as it is passing through O.
Torque at O due to 15 N force,
\[\tau_1 = 15 \times 6 \times {10}^{- 2} \times \sin37^\circ\]
\[ = 15 \times 6 \times {10}^{- 2} \times \frac{3}{5}\]
\[ = 0 . 54 N - m .........\left(\text{anticlockwise} \right)\]
Torque at O due to 10 N force,
\[\tau_2 = 10 \times 4 \times {10}^{- 2} = 0 . 4 N - m ........\left(\text{clockwise} \right)\]
Torque at O due to 20 N force,
\[\tau_3 = 20 \times 4 \times {10}^{- 2} \times \sin30^\circ\]
\[ = 20 \times 4 \times {10}^{- 2} \times \frac{1}{2}\]
\[ = 0 . 4 N - m .........\left(\text{Anticlockwise} \right)\]
Resultant torque acting at O,
\[\tau = \left( 0 . 54 - 0 . 4 + 0 . 4 \right)\]
\[ = 0 . 54 N - m \left(\text{Anticlockwise} \right)\]
APPEARS IN
RELATED QUESTIONS
A solid cylinder of mass 20 kg rotates about its axis with angular speed 100 rad s–1. The radius of the cylinder is 0.25 m. What is the kinetic energy associated with the rotation of the cylinder? What is the magnitude of the angular momentum of the cylinder about its axis?
The torque of a force \[\overrightarrow F \] about a point is defined as \[\overrightarrow\Gamma = \overrightarrow r \times \overrightarrow F.\] Suppose \[\overrightarrow r, \overrightarrow F\] and \[\overrightarrow \Gamma\] are all nonzero. Is \[r \times \overrightarrow\Gamma || \overrightarrow F\] always true? Is it ever true?
If several forces act on a particle, the total torque on the particle may be obtained by first finding the resultant force and then taking torque of this resultant. Prove this. Is this result valid for the forces acting on different particles of a body in such a way that their lines of action intersect at a common point?
If the resultant torque of all the forces acting on a body is zero about a point, is it necessary that it will be zero about any other point?
Equal torques act on the disc A and B of the previous problem, initially both being at rest. At a later instant, the linear speeds of a point on the rim of A and another point on the rim of B are \[\nu_A\] and \[\nu_B\] respectively. We have
A particle is moving with a constant velocity along a line parallel to the positive X-axis. The magnitude of its angular momentum with respect to the origin is, ______
The ratio of the acceleration for a solid sphere (mass m and radius R) rolling down an incline of angle θ without slipping and slipping down the incline without rolling is, ______
Define torque and mention its unit.
State conservation of angular momentum.
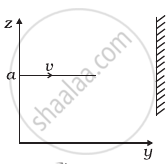
A particle of mass m is moving in yz-plane with a uniform velocity v with its trajectory running parallel to + ve y-axis and intersecting z-axis at z = a (Figure). The change in its angular momentum about the origin as it bounces elastically from a wall at y = constant is ______.
A Merry-go-round, made of a ring-like platform of radius R and mass M, is revolving with angular speed ω. A person of mass M is standing on it. At one instant, the person jumps off the round, radially away from the centre of the round (as seen from the round). The speed of the round afterwards is ______.
Figure shows two identical particles 1 and 2, each of mass m, moving in opposite directions with same speed v along parallel lines. At a particular instant, r1 and r2 are their respective position vectors drawn from point A which is in the plane of the parallel lines. Choose the correct options:
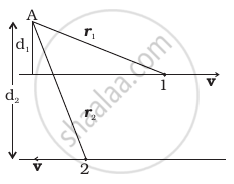
- Angular momentum l1 of particle 1 about A is l1 = mvd1
- Angular momentum l2 of particle 2 about A is l2 = mvr2
- Total angular momentum of the system about A is l = mv(r1 + r2)
- Total angular momentum of the system about A is l = mv (d2 − d1)
⊗ represents a unit vector coming out of the page.
⊗ represents a unit vector going into the page.
A uniform cube of mass m and side a is placed on a frictionless horizontal surface. A vertical force F is applied to the edge as shown in figure. Match the following (most appropriate choice):
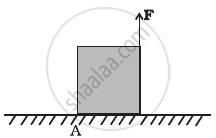
| (a) mg/4 < F < mg/2 | (i) Cube will move up. |
| (b) F > mg/2 | (ii) Cube will not exhibit motion. |
| (c) F > mg | (iii) Cube will begin to rotate and slip at A. |
| (d) F = mg/4 | (iv) Normal reaction effectively at a/3 from A, no motion. |
A door is hinged at one end and is free to rotate about a vertical axis (Figure). Does its weight cause any torque about this axis? Give reason for your answer.
A spherical shell of 1 kg mass and radius R is rolling with angular speed ω on horizontal plane (as shown in figure). The magnitude of angular momentum of the shell about the origin O is `a/3 R^2` ω. The value of a will be:
A solid sphere is rotating in free space. If the radius of the sphere is increased while keeping the mass the same, which one of the following will not be affected?
