Advertisements
Advertisements
प्रश्न
Evaluate the following: cos39° cos48° cos60° cosec42° cosec51°
उत्तर
cos39° cos48° cos60° cosec42° cosec51°
= `cos(90° - 51°) xx cos(90° - 42°) xx (1)/(2) xx (1)/(sin42°) xx (1)/(sin51°)`
= `sin51° xx sin42° xx (1)/(2) xx (1)/(sin42°) xx (1)/(sin51°)`
= `(1)/(2)`.
APPEARS IN
संबंधित प्रश्न
If 4 cos2 x° - 1 = 0 and 0 ∠ x° ∠ 90°,
find:(i) x°
(ii) sin2 x° + cos2 x°
(iii) `(1)/(cos^2xx°) – (tan^2 xx°)`
From the given figure,
find:
(i) cos x°
(ii) x°
(iii) `(1)/(tan^2 xx°) – (1)/(sin^2xx°)`
(iv) Use tan xo, to find the value of y.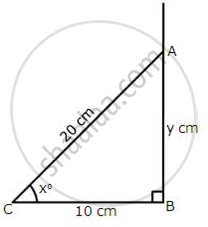
Find the magnitude of angle A, if 2 cos2 A - 3 cos A + 1 = 0
Solve for x : 3 tan2 (2x - 20°) = 1
Solve for x : cos2 30° + cos2 x = 1
Find the value of 'A', if (1 - cosec A)(2 - sec A) = 0
If `sqrt(2) = 1.414 and sqrt(3) = 1.732`, find the value of the following correct to two decimal places tan60°
In a rectangle ABCD, AB = 20cm, ∠BAC = 60°, calculate side BC and diagonals AC and BD.
Evaluate the following: `(2sin28°)/(cos62°) + (3cot49°)/(tan41°)`
Prove the following: sin230° + cos230° = `(1)/(2)sec60°`
