Advertisements
Advertisements
प्रश्न
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
f(x) = x2
उत्तर
Given function f(x) = x2
⇒ f'(x) = 2x
For maximum/minimum or critical points,
If f'(x) = 0 then 2x = 0 or x = 0
f'(x) changes sign from negative to positive as it passes through x = 0.
∴ f has local minimum at x = 0.
Local minimum = f(0) = 0
APPEARS IN
संबंधित प्रश्न
Examine the maxima and minima of the function f(x) = 2x3 - 21x2 + 36x - 20 . Also, find the maximum and minimum values of f(x).
If `f'(x)=k(cosx-sinx), f'(0)=3 " and " f(pi/2)=15`, find f(x).
Find the maximum and minimum value, if any, of the following function given by f(x) = −(x − 1)2 + 10
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
`h(x) = sinx + cosx, 0 < x < pi/2`
Find the absolute maximum value and the absolute minimum value of the following function in the given interval:
f (x) = (x −1)2 + 3, x ∈[−3, 1]
Find both the maximum value and the minimum value of 3x4 − 8x3 + 12x2 − 48x + 25 on the interval [0, 3].
Show that semi-vertical angle of right circular cone of given surface area and maximum volume is `Sin^(-1) (1/3).`
A window is in the form of a rectangle surmounted by a semicircular opening. The total perimeter of the window is 10 m. Find the dimensions of the window to admit maximum light through the whole opening
Find the absolute maximum and minimum values of the function f given by f (x) = cos2 x + sin x, x ∈ [0, π].
Find the point on the straight line 2x+3y = 6, which is closest to the origin.
Find the maximum and minimum of the following functions : f(x) = x3 – 9x2 + 24x
Find the maximum and minimum of the following functions : f(x) = x log x
A ball is thrown in the air. Its height at any time t is given by h = 3 + 14t – 5t2. Find the maximum height it can reach.
Show that among rectangles of given area, the square has least perimeter.
Find the volume of the largest cylinder that can be inscribed in a sphere of radius ‘r’ cm.
Solve the following:
A rectangular sheet of paper of fixed perimeter with the sides having their lengths in the ratio 8 : 15 converted into an open rectangular box by folding after removing the squares of equal area from all corners. If the total area of the removed squares is 100, the resulting box has maximum volume. Find the lengths of the rectangular sheet of paper.
A metal wire of 36cm long is bent to form a rectangle. Find it's dimensions when it's area is maximum.
By completing the following activity, examine the function f(x) = x3 – 9x2 + 24x for maxima and minima
Solution: f(x) = x3 – 9x2 + 24x
∴ f'(x) = `square`
∴ f''(x) = `square`
For extreme values, f'(x) = 0, we get
x = `square` or `square`
∴ f''`(square)` = – 6 < 0
∴ f(x) is maximum at x = 2.
∴ Maximum value = `square`
∴ f''`(square)` = 6 > 0
∴ f(x) is maximum at x = 4.
∴ Minimum value = `square`
The minimum value of Z = 5x + 8y subject to x + y ≥ 5, 0 ≤ x ≤ 4, y ≥ 2, x ≥ 0, y ≥ 0 is ____________.
Find all the points of local maxima and local minima of the function f(x) = `- 3/4 x^4 - 8x^3 - 45/2 x^2 + 105`
Let f have second derivative at c such that f′(c) = 0 and f"(c) > 0, then c is a point of ______.
An open box with square base is to be made of a given quantity of cardboard of area c2. Show that the maximum volume of the box is `"c"^3/(6sqrt(3))` cubic units
A metal box with a square base and vertical sides is to contain 1024 cm3. The material for the top and bottom costs Rs 5/cm2 and the material for the sides costs Rs 2.50/cm2. Find the least cost of the box.
The function f(x) = 2x3 – 3x2 – 12x + 4, has ______.
Find all the points of local maxima and local minima of the function f(x) = (x - 1)3 (x + 1)2
Find both the maximum and minimum values respectively of 3x4 - 8x3 + 12x2 - 48x + 1 on the interval [1, 4].
The function `f(x) = x^3 - 6x^2 + 9x + 25` has
A wire of length 22 m is to be cut into two pieces. One of the pieces is to be made into a square and the other into an equilateral triangle. Then, the length of the side of the equilateral triangle, so that the combined area of the square and the equilateral triangle is minimum, is ______.
If S1 and S2 are respectively the sets of local minimum and local maximum points of the function. f(x) = 9x4 + 12x3 – 36x2 + 25, x ∈ R, then ______.
If the function y = `(ax + b)/((x - 4)(x - 1))` has an extremum at P(2, –1), then the values of a and b are ______.
Let f(x) = (x – a)ng(x) , where g(n)(a) ≠ 0; n = 0, 1, 2, 3.... then ______.
A rod AB of length 16 cm. rests between the wall AD and a smooth peg, 1 cm from the wall and makes an angle θ with the horizontal. The value of θ for which the height of G, the midpoint of the rod above the peg is minimum, is ______.
Read the following passage:
Engine displacement is the measure of the cylinder volume swept by all the pistons of a piston engine. The piston moves inside the cylinder bore.
|
Based on the above information, answer the following questions:
- If the radius of cylinder is r cm and height is h cm, then write the volume V of cylinder in terms of radius r. (1)
- Find `(dV)/(dr)`. (1)
- (a) Find the radius of cylinder when its volume is maximum. (2)
OR
(b) For maximum volume, h > r. State true or false and justify. (2)
The rectangle has area of 50 cm2. Complete the following activity to find its dimensions for least perimeter.
Solution: Let x cm and y cm be the length and breadth of a rectangle.
Then its area is xy = 50
∴ `y =50/x`
Perimeter of rectangle `=2(x+y)=2(x+50/x)`
Let f(x) `=2(x+50/x)`
Then f'(x) = `square` and f''(x) = `square`
Now,f'(x) = 0, if x = `square`
But x is not negative.
∴ `x = root(5)(2) "and" f^('')(root(5)(2))=square>0`
∴ by the second derivative test f is minimum at x = `root(5)(2)`
When x = `root(5)(2),y=50/root(5)(2)=root(5)(2)`
∴ `x=root(5)(2) "cm" , y = root(5)(2) "cm"`
Hence, rectangle is a square of side `root(5)(2) "cm"`
Find the maximum and the minimum values of the function f(x) = x2ex.
Sumit has bought a closed cylindrical dustbin. The radius of the dustbin is ‘r' cm and height is 'h’ cm. It has a volume of 20π cm3.
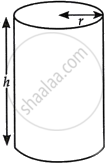
- Express ‘h’ in terms of ‘r’, using the given volume.
- Prove that the total surface area of the dustbin is `2πr^2 + (40π)/r`
- Sumit wants to paint the dustbin. The cost of painting the base and top of the dustbin is ₹ 2 per cm2 and the cost of painting the curved side is ₹ 25 per cm2. Find the total cost in terms of ‘r’, for painting the outer surface of the dustbin including the base and top.
- Calculate the minimum cost for painting the dustbin.



