Advertisements
Advertisements
प्रश्न
Find the point on the X–axis which is equidistant from A(–3, 4) and B(1, –4).
उत्तर
Let point C be on the X-axis which is equidistant from points A and B.
Point C lies on X-axis.
∴ Its y co-ordinate is 0.
Let C = (x, 0)
C is equidistant from points A and B.
∴ AC = BC
According to distance formula,
`∴ sqrt([x – (– 3)]^2 + (0 – 4)^2] = sqrt((x – 1)^2 + [0 – ( – 4)]^2)`
∴ [x – (– 3)]2 + (0 – 4)2 = (x – 1)2 + [0 – ( – 4)]2 ...(Squaring both the sides)
∴ (x + 3)2 + (– 4)2 = (x – 1)2 + 42
∴ x2 + 6x + 9 + 16 = x2 – 2x + 1 + 16
∴ `cancelx^2 + 6x + 25 = cancelx^2 – 2x + 17`
∴ 6x + 2x = 17 – 25
∴ 8x = – 8
∴ `"x" = (-8)/8`
∴ x = –1
∴ The point on the X-axis, which is equidistant from points A and B, is (–1, 0).
APPEARS IN
संबंधित प्रश्न
Write the equation of the line passing through the pair of points (2, 3) and (4, 7) in the form of y = mx + c.
If (4,-3) is a point on the line AB and slope of the line is (-2), write the equation of the line AB.
Write the equation of each of the following lines:
- The x-axis and the y-axis.
- The line passing through the origin and the point (-3, 5).
- The line passing through the point (-3, 4) and parallel to X-axis.
In ΔABC, A(3, 5), B(7, 8) and C(1, –10). Find the equation of the median through A.
Find the slope and y-intercept of the line:
3x – 4y = 5
The equation of a line is x – y = 4. Find its slope and y-intercept. Also, find its inclination.
Find the equation of the line passing through (−2, 1) and perpendicular to 4x + 5y = 6.
Find the equation of the perpendicular bisector of the line segment obtained on joining the points (6, −3) and (0, 3).
The line 4x − 3y + 12 = 0 meets x-axis at A. Write the co-ordinates of A. Determine the equation of the line through A and perpendicular to 4x – 3y + 12 = 0.
The point P is the foot of perpendicular from A(−5, 7) to the line whose equation is 2x – 3y + 18 = 0. Determine :
- the equation of the line AP.
- the co-ordinates of P.
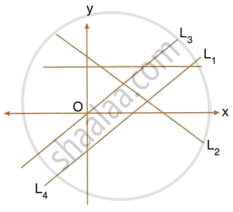
Match the equations A, B, C and D with the lines L1, L2, L3 and L4, whose graphs are roughly drawn in the given diagram.
A ≡ y = 2x;
B ≡ y – 2x + 2 = 0;
C ≡ 3x + 2y = 6;
D ≡ y = 2
Find the equation of the line which is perpendicular to the line `x/a - y/b = 1` at the point where this line meets y-axis.
Verify that points P(–2, 2), Q(2, 2) and R(2, 7) are vertices of a right angled triangle.
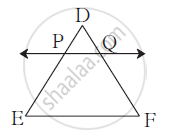
In Δ DEF, line PQ || side EF, If DP = 2.4,
PE = 7.2, DQ = 1 then find QF.
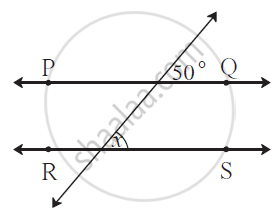
In the figure, line PQ || line RS. Using the information given
in the figure find the value of x.
A straight line passes through the points P(–1, 4) and Q(5, –2). It intersects x-axis at point A and y-axis at point B. M is the mid-point of the line segment AB. Find:
- the equation of the line.
- the co-ordinates of point A and B.
- the co-ordinates of point M.
Three vertices of a parallelogram ABCD taken in order are A(3, 6), B(5, 10) and C(3, 2), find:
- the co-ordinates of the fourth vertex D.
- length of diagonal BD.
- equation of side AB of the parallelogram ABCD.
Find the equation of the line passing through the points (4,-5) and (-1,-2).
