Advertisements
Advertisements
प्रश्न
Identify the type of conic and find centre, foci, vertices, and directrices of the following:
`x^2/25 - y^2/144` = 1
उत्तर
It is Hyperbola.
The transverse axis the x-axis.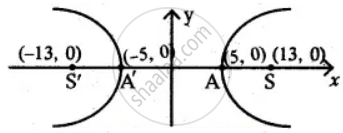
a2 = 25, b2 = 144
a = 5, b = 12
c2 = a2 + b2
= 25 + 144
= 169
c = 13
ae = 13
5e = 13
e = `13/5`
(a) Centre (0, 0)
(b) Vertex (± a, 0) = (± 5, 0)
(c) Foci (± c, 0) = (± 13, 0)
(d) Equation of the directrix
x = `+- "a"/"e" = +- 5/(13/5) = +- 25/13`
x = `+- 25/13`
APPEARS IN
संबंधित प्रश्न
The parabola y2 = kx passes through the point (4, -2). Find its latus rectum and focus.
Find the co-ordinates of the focus, vertex, equation of the directrix, axis and the length of latus rectum of the parabola
y2 = 20x
Find the co-ordinates of the focus, vertex, equation of the directrix, axis and the length of latus rectum of the parabola
x2 = 8y
The profit ₹ y accumulated in thousand in x months is given by y = -x2 + 10x – 15. Find the best time to end the project.
Find the equation of the parabola which is symmetrical about x-axis and passing through (–2, –3).
The focus of the parabola x2 = 16y is:
The eccentricity of the parabola is:
Find the equation of the parabola in the cases given below:
Focus (4, 0) and directrix x = – 4
Find the equation of the ellipse in the cases given below:
Foci `(+- 3, 0), "e"+ 1/2`
Find the equation of the hyperbola in the cases given below:
Centre (2, 1), one of the foci (8, 1) and corresponding directrix x = 4
Find the equation of the hyperbola in the cases given below:
Passing through (5, – 2) and length of the transverse axis along x-axis and of length 8 units
Find the vertex, focus, equation of directrix and length of the latus rectum of the following:
y2 = 16x
Identify the type of conic and find centre, foci, vertices, and directrices of the following:
`x^2/25 + y^2/9` = 1
Show that the absolute value of difference of the focal distances of any point P on the hyperbola is the length of its transverse axis
Identify the type of conic and find centre, foci, vertices, and directrices of the following:
`(x - 3)^2/225 + (y - 4)^2/289` = 1
Identify the type of conic and find centre, foci, vertices, and directrices of the following:
`(x + 3)^2/225 + (y - 4)^2/64` = 1
Identify the type of conic and find centre, foci, vertices, and directrices of the following:
`(y - 2)^3/25 + (x + 1)^2/16` = 1
Choose the correct alternative:
If P(x, y) be any point on 16x2 + 25y2 = 400 with foci F(3, 0) then PF1 + PF2 is
