Advertisements
Advertisements
प्रश्न
Identify the type of conic and find centre, foci, vertices, and directrices of the following:
`y^2/16 - x^2/9` = 1
उत्तर
It is Hyperbola.
The transverse axis the y axis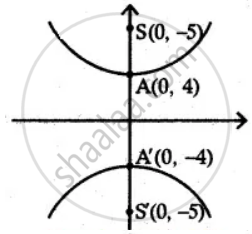
a2 = 16, b2 = 9
a = 4, b = 3
c2 = a2 + b2
= 16 + 6 = 25
c = 5
ae = 5
4e = 5
e = `5/4`
(a) Centre (0, 0)
(b) Vertex (0, ± a) = (0, ± 4)
(c) Foci (0, ± ae) = (0, ± 5)
(d) Equation of the directrix
y = `+- "a"/"e" = +- 4/(5/4) = +- 16/5`
y = `+- 16/5`
APPEARS IN
संबंधित प्रश्न
Find the equation of the parabola whose focus is the point F(-1, -2) and the directrix is the line 4x – 3y + 2 = 0.
Find the co-ordinates of the focus, vertex, equation of the directrix, axis and the length of latus rectum of the parabola
x2 = - 16y
The average variable cost of the monthly output of x tonnes of a firm producing a valuable metal is ₹ `1/5`x2 – 6x + 100. Show that the average variable cost curve is a parabola. Also, find the output and the average cost at the vertex of the parabola.
Find the equation of the parabola which is symmetrical about x-axis and passing through (–2, –3).
The distance between directrix and focus of a parabola y2 = 4ax is:
Find the equation of the parabola in the cases given below:
Passes through (2, – 3) and symmetric about y-axis
Find the equation of the parabola in the cases given below:
Vertex (1, – 2) and Focus (4, – 2)
Find the equation of the ellipse in the cases given below:
Foci `(+- 3, 0), "e"+ 1/2`
Find the equation of the ellipse in the cases given below:
Length of latus rectum 4, distance between foci `4sqrt(2)`, centre (0, 0) and major axis as y-axis
Find the equation of the hyperbola in the cases given below:
Foci (± 2, 0), Eccentricity = `3/2`
Find the equation of the hyperbola in the cases given below:
Passing through (5, – 2) and length of the transverse axis along x-axis and of length 8 units
Find the vertex, focus, equation of directrix and length of the latus rectum of the following:
y2 = 16x
Find the vertex, focus, equation of directrix and length of the latus rectum of the following:
x2 = 24y
Find the vertex, focus, equation of directrix and length of the latus rectum of the following:
y2 = – 8x
Find the vertex, focus, equation of directrix and length of the latus rectum of the following:
y2 – 4y – 8x + 12 = 0
Prove that the length of the latus rectum of the hyperbola `x^2/"a"^2 - y^2/"b"^2` = 1 is `(2"b"^2)/"a"`
Show that the absolute value of difference of the focal distances of any point P on the hyperbola is the length of its transverse axis
Identify the type of conic and find centre, foci, vertices, and directrices of the following:
`(x + 1)^2/100 + (y - 2)^2/64` = 1
Choose the correct alternative:
If P(x, y) be any point on 16x2 + 25y2 = 400 with foci F(3, 0) then PF1 + PF2 is
