Advertisements
Advertisements
Question
Identify the type of conic and find centre, foci, vertices, and directrices of the following:
`y^2/16 - x^2/9` = 1
Solution
It is Hyperbola.
The transverse axis the y axis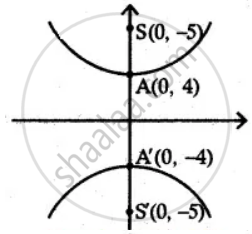
a2 = 16, b2 = 9
a = 4, b = 3
c2 = a2 + b2
= 16 + 6 = 25
c = 5
ae = 5
4e = 5
e = `5/4`
(a) Centre (0, 0)
(b) Vertex (0, ± a) = (0, ± 4)
(c) Foci (0, ± ae) = (0, ± 5)
(d) Equation of the directrix
y = `+- "a"/"e" = +- 4/(5/4) = +- 16/5`
y = `+- 16/5`
APPEARS IN
RELATED QUESTIONS
Find the equation of the parabola whose focus is the point F(-1, -2) and the directrix is the line 4x – 3y + 2 = 0.
The parabola y2 = kx passes through the point (4, -2). Find its latus rectum and focus.
Find the vertex, focus, axis, directrix, and the length of the latus rectum of the parabola y2 – 8y – 8x + 24 = 0.
Find the co-ordinates of the focus, vertex, equation of the directrix, axis and the length of latus rectum of the parabola
x2 = - 16y
The average variable cost of the monthly output of x tonnes of a firm producing a valuable metal is ₹ `1/5`x2 – 6x + 100. Show that the average variable cost curve is a parabola. Also, find the output and the average cost at the vertex of the parabola.
Find the axis, vertex, focus, equation of directrix and the length of latus rectum of the parabola (y - 2)2 = 4(x - 1)
The eccentricity of the parabola is:
Find the equation of the parabola in the cases given below:
Passes through (2, – 3) and symmetric about y-axis
Find the equation of the ellipse in the cases given below:
Length of latus rectum 8, eccentricity = `3/5` centre (0, 0) and major axis on x-axis
Find the vertex, focus, equation of directrix and length of the latus rectum of the following:
y2 = 16x
Find the vertex, focus, equation of directrix and length of the latus rectum of the following:
x2 = 24y
Find the vertex, focus, equation of directrix and length of the latus rectum of the following:
y2 = – 8x
Identify the type of conic and find centre, foci, vertices, and directrices of the following:
`x^2/25 + y^2/9` = 1
Identify the type of conic and find centre, foci, vertices, and directrices of the following:
`x^2/3 + y^2/10` = 1
Prove that the length of the latus rectum of the hyperbola `x^2/"a"^2 - y^2/"b"^2` = 1 is `(2"b"^2)/"a"`
Show that the absolute value of difference of the focal distances of any point P on the hyperbola is the length of its transverse axis
