Samacheer Kalvi solutions for Mathematics - Volume 1 and 2 [English] Class 12 TN Board chapter 5 - Two Dimensional Analytical Geometry-II [Latest edition]

Obtain the equation of the circles with radius 5 cm and touching x-axis at the origin in general form

Find the equation of the circle with centre (2, −1) and passing through the point (3, 6) in standard form

Find the equation of circles that touch both the axes and pass through (− 4, −2) in general form

Find the equation of the circles with centre (2, 3) and passing through the intersection of the lines 3x – 2y – 1 = 0 and 4x + y – 27 = 0

Obtain the equation of the circle for which (3, 4) and (2, -7) are the ends of a diameter.

Find the equation of the circle through the points (1, 0), (– 1, 0) and (0, 1)

A circle of area 9π square units has two of its diameters along the lines x + y = 5 and x – y = 1. Find the equation of the circle

If y = `2sqrt(2)x + "c"` is a tangent to the circle x² + y² = 16, find the value of c

Find the equation of the tangent and normal to the circle x² + y² – 6x + 6y – 8 = 0 at (2, 2)

Determine whether the points (– 2, 1), (0, 0) and (– 4, – 3) lie outside, on or inside the circle x² + y² – 5x + 2y – 5 = 0

Find centre and radius of the following circles

x² + (y + 2)² = 0

Find centre and radius of the following circles

x² + y² + 6x – 4y + 4 = 0

Find centre and radius of the following circles

x² + y² – x + 2y – 3 = 0

Find centre and radius of the following circles

2x² + 2y² – 6x + 4y + 2 = 0

If the equation 3x² + (3 – p)xy + qy² – 2px = 8pq represents a circle, find p and q. Also determine the centre and radius of the circle

Find the equation of the parabola in the cases given below:

Focus (4, 0) and directrix x = – 4

Find the equation of the parabola in the cases given below:

Passes through (2, – 3) and symmetric about y-axis

Find the equation of the parabola in the cases given below:

Vertex (1, – 2) and Focus (4, – 2)

Find the equation of the parabola in the cases given below:

End points of latus rectum (4, – 8) and (4, 8)

Find the equation of the ellipse in the cases given below:

Foci `(+- 3, 0), "e"+ 1/2`

Find the equation of the ellipse in the cases given below:

Foci (0, ±4) and end points of major axis are (0, ±5)

Find the equation of the ellipse in the cases given below:

Length of latus rectum 8, eccentricity = `3/5` centre (0, 0) and major axis on x-axis

Find the equation of the ellipse in the cases given below:

Length of latus rectum 4, distance between foci `4sqrt(2)`, centre (0, 0) and major axis as y-axis

Find the equation of the hyperbola in the cases given below:

Foci (± 2, 0), Eccentricity = `3/2`

Find the equation of the hyperbola in the cases given below:

Centre (2, 1), one of the foci (8, 1) and corresponding directrix x = 4

Find the equation of the hyperbola in the cases given below:

Passing through (5, – 2) and length of the transverse axis along x-axis and of length 8 units

Find the vertex, focus, equation of directrix and length of the latus rectum of the following:

y² = 16x

Find the vertex, focus, equation of directrix and length of the latus rectum of the following:

x² = 24y

Find the vertex, focus, equation of directrix and length of the latus rectum of the following:

y² = – 8x

Find the vertex, focus, equation of directrix and length of the latus rectum of the following:

x² – 2x + 8y + 17 = 0

Find the vertex, focus, equation of directrix and length of the latus rectum of the following:

y² – 4y – 8x + 12 = 0

Identify the type of conic and find centre, foci, vertices, and directrices of the following:

`x^2/25 + y^2/9` = 1

Identify the type of conic and find centre, foci, vertices, and directrices of the following:

`x^2/3 + y^2/10` = 1

Identify the type of conic and find centre, foci, vertices, and directrices of the following:

`x^2/25 - y^2/144` = 1

Identify the type of conic and find centre, foci, vertices, and directrices of the following:

`y^2/16 - x^2/9` = 1

Prove that the length of the latus rectum of the hyperbola `x^2/"a"^2 - y^2/"b"^2` = 1 is `(2"b"^2)/"a"`

Show that the absolute value of difference of the focal distances of any point P on the hyperbola is the length of its transverse axis

Identify the type of conic and find centre, foci, vertices, and directrices of the following:

`(x - 3)^2/225 + (y - 4)^2/289` = 1

Identify the type of conic and find centre, foci, vertices, and directrices of the following:

`(x + 1)^2/100 + (y - 2)^2/64` = 1

Identify the type of conic and find centre, foci, vertices, and directrices of the following:

`(x + 3)^2/225 + (y - 4)^2/64` = 1

Identify the type of conic and find centre, foci, vertices, and directrices of the following:

`(y - 2)^3/25 + (x + 1)^2/16` = 1

Identify the type of conic and find centre, foci, vertices, and directrices of the following:

18x² + 12y² – 144x + 48y + 120 = 0

Identify the type of conic and find centre, foci, vertices, and directrices of the following:

9x² – y² – 36x – 6y + 18 = 0

Identify the type of conic section for the equation.

2x² – y² = 7

Identify the type of conic section for the equation.

3x² + 3y² – 4x + 3y + 10 = 0

Identify the type of conic section for the equation.

3x² + 2y² = 14

Identify the type of conic section for the equation.

x² + y² + x – y = 0

Identify the type of conic section for the equation.

11x² – 25y² – 44x + 50y – 256 = 0

Identify the type of conic section for the equation.

y² + 4x + 3y + 4 = 0

Find the equations of the two tangents that can be drawn from (5, 2) to the ellipse 2x² + 7y² = 14

Find the equations of tangents to the hyperbola `x^2/16 - y^2/64` = 1 which are parallel to10x − 3y + 9 = 0

Show that the line x – y + 4 = 0 is a tangent to the ellipse x² + 3y² = 12. Also find the coordinates of the point of contact

Find the equation of the tangent to the parabola y² = 16x perpendicular to 2x + 2y + 3 = 0

Find the equation of the tangent at t = 2 to the parabola y² = 8x (Hint: use parametric form)

Find the equations of the tangent and normal to hyperbola 12x² – 9y² = 108 at θ = `pi/3`. (Hint: use parametric form)

Prove that the point of intersection of the tangents at ‘t₁‘ and t₂’ on the parabola y² = 4ax is [at₁ t₂, a (t₁ + t₂)]

If the normal at the point ‘t₁‘ on the parabola y² = 4ax meets the parabola again at the point ‘t₂‘, then prove that t₂ = `- ("t"_1 + 2/"t"_1)`

A bridge has a parabolic arch that is 10 m high in the centre and 30 m wide at the bottom. Find the height of the arch 6m from the centre, on either sides

A tunnel through a mountain for a four-lane highway is to have a elliptical opening. The total width of the highway (not the opening) is to be 16 m, and the height at the edge of the road must be sufficient for a truck 4 m high to clear if the highest point of the opening is to be 5 m approximately. How wide must the opening be?

At a water fountain, water attains a maximum height of 4 m at horizontal distance of 0.5 m from its origin. If the path of water is a parabola, find the height of water at a horizontal distance of 0.75 m from the point of origin.

An engineer designs a satellite dish with a parabolic cross-section. The dish is  5m wide at the opening, and the focus is placed 1 2. m from the vertex. Position a coordinate system with the origin at the vertex and the x-axis on the parabola’s axis of symmetry and find an equation of the parabola

An engineer designs a satellite dish with a parabolic cross-section. The dish is 5 m wide at the opening and the focus is placed 1.2 m from the vertex. Find the depth of the satellite dish at the vertex

Parabolic cable of a 60 m portion of the roadbed of a suspension bridge are positioned as shown below. Vertical Cables are to be spaced every 6m along this portion of the roadbed. Calculate the lengths of first two of these vertical cables from the vertex.

Cross-section of a Nuclear cooling tower is in the shape of a hyperbola with equation `x^2/30^2 - y^2/44^2` = 1. The tower is 150 m tall and the distance from the top of the tower to the centre of the hyperbola is half the distance from the base of the tower to the centre of the hyperbola. Find the diameter of the top and base of the tower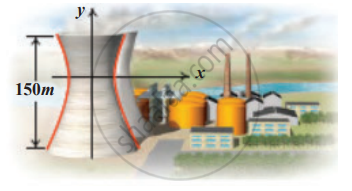

A rod of length 1 2. m moves with its ends always touching the coordinate axes. The locus of a point P on the rod, which is 0 3. m from the end in contact with x-axis is an ellipse. Find the eccentricity

Assume that water issuing from the end of a horizontal pipe, 7 5. m above the ground, describes a parabolic path. The vertex of the parabolic path is at the end of the pipe. At a position 2 5. m below the line of the pipe, the flow of water has curved outward 3 m beyond the vertical line through the end of the pipe. How far beyond this vertical line will the water strike the ground?

On lighting a rocket cracker it gets projected in a parabolic path and reaches a maximum height of 4 m when it is 6m away from the point of projection. Finally it reaches the ground 12 m away from the starting point. Find the angle of projection

Points A and B are 10 km apart and it is determined from the sound of an explosion heard at those points at different times that the location of the explosion is 6 km closer to A than B. Show that the location of the explosion is restricted to a particular curve and find an equation of it.

Choose the correct alternative:

The equation of the circle passing through (1, 5) and (4, 1) and touching y-axis `x^2 + y^2 - 5x - 6y + 9 + lambda(4x + 3y - 19)` = where `lambda` is equal to

Choose the correct alternative:

The eccentricity of the hyperbola whose latus rectum is 8 and conjugate axis is equal to half the distance between the foci is

Choose the correct alternative:

The circle x² + y² = 4x + 8y + 5 intersects the line 3x – 4y = m at two distinct points if

Choose the correct alternative:

The length of the diameter of the circle which touches the x -axis at the point (1, 0) and passes through the point (2, 3)

Choose the correct alternative:

The radius of the circle 3x² + by² + 4bx – 6by + b² = 0 is

Choose the correct alternative:

The centre of the circle inscribed in a square formed by the lines `x^2 - 8x - 12` = 0 and `y^2 - 14y + 45` = 0 is

Choose the correct alternative:

The equation of the normal to the circle x² + y² – 2x – 2y + 1 = 0 which is parallel to the line 2x + 4y = 3 is

Choose the correct alternative:

If P(x, y) be any point on 16x² + 25y² = 400 with foci F(3, 0) then PF₁ + PF₂ is

Choose the correct alternative:

The radius of the circle passing through the points (6, 2) two of whose diameter are x + y = 6 and x + 2y = 4 is

Choose the correct alternative:

The area of quadrilateral formed with foci of the hyperbolas `x^2/"a"^2 - y^2/"b"^2` = 1 and `1x^2/"a"^2 - y^2/"b"^2` = – 1

Choose the correct alternative:

If the normals of the parabola y² = 4x drawn at the end points of its latus rectum are tangents to the circle (x – 3)² + (y + 2)² = r², then the value of r² is

Choose the correct alternative:

If x + y = k is a normal to the parabola y² = 12x, then the value of k is 14

Choose the correct alternative:

The ellipse E₁ : `x^2/9 + y^2/4` = 1 is inscribed in a rectangle R whose sides are parallel to the co-ordinate axes. Another ellipse E₂ passing through the point (0, 4) circumscribes the rectangle R. The eccentricity of the ellipse is

Choose the correct alternative:

Tangents are drawn to, the, hyperbola `x^2/9 - y^2/4` = 1 parallel to the straight line 2x – y – 1. One of the points of contact of tangents on the hyperbola is

Choose the correct alternative:

The equation of the circle passing through the foci of the ellipse `x^2/16 +  y^2/9` = 1 having centre at (0, 3) is

Choose the correct alternative:

Let C be the circle with centre at (1, 1) and radius = 1. If T is the circle centered at (0, y) passing through the origin and touching the circle C externally, then the radius of T is equal to

Choose the correct alternative:

Consider an ellipse whose centre is of the origin and its major axis is a long x-axis. If its eccentricity is `3/5` and the distance between its foci is 6, then the area of the quadrilateral’ inscribed in the ellipse with diagonals as major and minor axis, of the ellipse is

Choose the correct alternative:

Area of the greatest rectangle inscribed in the ellipse `x^2/"a"^2 + y^2/"b"^2` = 1 is

Choose the correct alternative:

An ellipse has OB as semi-minor axes, F and F’ its foci and the angle FBF’ is a right angle. Then the eccentricity of the ellipse is

Choose the correct alternative:

The eccentricity of the ellipse (x – 3)² + (y – 4)² = `y^2/9` is

Choose the correct alternative:

If the two tangents drawn from a point P to the parabola y² = 4r are at right angles then the locus of P is 

Choose the correct alternative:

The circle passing through (1, – 2) and touching the axis of x at (3, 0) passing through the point

Choose the correct alternative:

The locus of a point whose distance from (– 2, 0) is `2/3` times its distance from the line x = `(-9)/2` is

Choose the correct alternative:

The values of m for which the line y = `"m"x + 2sqrt(5)` touches the hyperbola 16x² – 9y² = 144 are the roots of x² – (a + b)x – 4 = 0, then the value of (a + b) is

Choose the correct alternative:

If the coordinates at one end of a diameter of the circle x² + y² – 8x – 4y + c = 0 are (11, 2) the coordinates of the other end are