Advertisements
Advertisements
प्रश्न
If ∆ABC ∼ ∆DEF such that DE = 3 cm, EF = 2 cm, DF = 2.5 cm, BC = 4 cm, then perimeter of ∆ABC is
पर्याय
18 cm
20 cm
12 cm
15 cm
उत्तर
Given: ΔABC and ΔDEF are similar triangles such that DE = 3cm, EF = 2cm, DF = 2.5cm and BC = 4cm.
To find: Perimeter of ΔABC.
We know that if two triangles are similar then their corresponding sides are proportional.
Hence, `(AB)/(DE)=(BC)/(EF)=(CA)/(FD)`
Substituting the values we get
`(AB)/(BC)=(DE)/(EF)`
`(AB)/4=3/2`
`AB=6cm`..................(1)
Similarly,
`(CA)/(BE)=(DF)/(EF)`
`(CA)/4=2.5/2`
`CA=5cm`
Perimeter of Δ ABC = AB+BC+CA
= 6+4+5
= 15 cm
Hence the correct option is `d`
APPEARS IN
संबंधित प्रश्न
ABCD is a quadrilateral in which AD = BC. If P, Q, R, S be the mid-points of AB, AC, CD and BD respectively, show that PQRS is a rhombus.
In a ΔABC, AD is the bisector of ∠A.
If AB = 6.4cm, AC = 8cm and BD = 5.6cm, find DC.
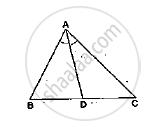
In a ΔABC, AD is the bisector of ∠A.
If AB = 5.6cm, AC = 4cm and DC = 3cm, find BC.

In ∆ABC, ∠ABC = 135°. Prove that AC2 = AB2 + BC2 + 4 ar (∆ABC)
State Pythagoras theorem and its converse.
If ∆ABC and ∆DEF are two triangles such tha\[\frac{AB}{DE} = \frac{BC}{EF} = \frac{CA}{FD} = \frac{2}{5}\] , then Area (∆ABC) : Area (∆DEF) =
In triangles ABC and DEF, ∠A = ∠E = 40°, AB : ED = AC : EF and ∠F = 65°, then ∠B =
If D, E, F are the mid-points of sides BC, CA and AB respectively of ∆ABC, then the ratio of the areas of triangles DEF and ABC is
The areas of two similar triangles are 121 cm2 and 64 cm2 respectively. If the median of the first triangle is 12.1 cm, then the corresponding median of the other triangle is
If ABC is an isosceles triangle and D is a point of BC such that AD ⊥ BC, then
