Advertisements
Advertisements
प्रश्न
In a circle, the major arc is 3 times the minor arc. The corresponding central angles and the degree measures of two arcs are
पर्याय
90° and 270°
90° and 90°
270° and 90°
60° and 210°
उत्तर
270° and 90°
We are given the major arc is 3 times the minor arc. We are asked to find the corresponding central angle.
See the corresponding figure.

We know that angle formed by the circumference at the centre is 360°.
Since the circumference of the circle is divided into two parts such that the angle formed by major and minor arcs at the centre are 3x and x respectively.
So 3x + x = 360
4x = 360
x = 90
So m \[\stackrel\frown{AB}\] = 90° and m \[\stackrel\frown{AB}\] = 3x = 270°
APPEARS IN
संबंधित प्रश्न
Fill in the blank:
Segment of a circle is the region between an arc and .................. of the circle.
If O is the centre of the circle, find the value of x in the following figure:

If O is the centre of the circle, find the value of x in the following figure:
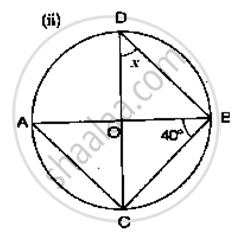
If O is the centre of the circle, find the value of x in the following figures.

O is the circumcentre of the triangle ABC and OD is perpendicular on BC. Prove that ∠BOD = ∠A
In the given figure, O is the centre of the circle, prove that ∠x = ∠y + ∠z.
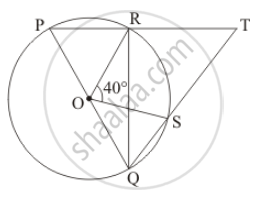
In the given figure, O is the centre of a circle and PQ is a diameter. If ∠ROS = 40°, find ∠RTS.
If ABC is an arc of a circle and ∠ABC = 135°, then the ratio of arc \[\stackrel\frown{ABC}\] to the circumference is ______.
The chord of a circle is equal to its radius. The angle subtended by this chord at the minor arc of the circle is
A circle has radius `sqrt(2)` cm. It is divided into two segments by a chord of length 2 cm. Prove that the angle subtended by the chord at a point in major segment is 45°.
