Advertisements
Advertisements
प्रश्न
In the given figure, if O is the circumcentre of ∠ABC, then find the value of ∠OBC + ∠BAC.
Advertisements
उत्तर
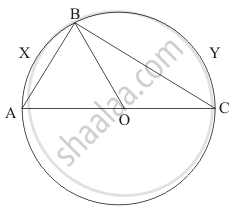
Since, O is the circumcentre of \[\bigtriangleup ABC\], So, O would be centre of the circle passing through points A, B and C.
As OA = OB (Radii of the same circle)
\[\therefore \angle OAB = \angle OBA \left( \text{ Angle opposite to equal sides are equal } \right)\]
\[\text{ or } , \angle BAC = \angle OBA\]
\[\text{ From } \left( 1 \right)\]
\[\angle BAC + \angle OBC = 90°\]
APPEARS IN
संबंधित प्रश्न
Fill in the blank:
Segment of a circle is the region between an arc and .................. of the circle.
Given an arc of a circle, show how to complete the circle.
In the below fig. O is the centre of the circle. Find ∠BAC.
O is the circumcentre of the triangle ABC and OD is perpendicular on BC. Prove that ∠BOD = ∠A.
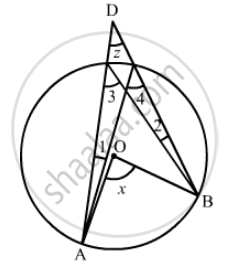
In the given figure, O is the centre of the circle, BO is the bisector of ∠ABC. Show that AB = BC.
In the given figure, O and O' are centres of two circles intersecting at B and C. ACD is a straight line, find x.
In the given figure, O is the centre of a circle and PQ is a diameter. If ∠ROS = 40°, find ∠RTS.
In the given figure, P and Q are centres of two circles intersecting at B and C. ACD is a straight line. Then, ∠BQD =
In a circle, the major arc is 3 times the minor arc. The corresponding central angles and the degree measures of two arcs are
In the following figure, AB and CD are two chords of a circle intersecting each other at point E. Prove that ∠AEC = `1/2` (Angle subtended by arc CXA at centre + angle subtended by arc DYB at the centre).
