Advertisements
Advertisements
प्रश्न
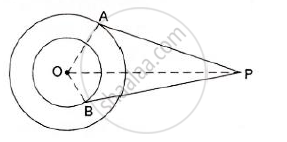
In the given figure, O is the centre of the two concentric circles of radii 4 cm and 6cm respectively. AP and PB are tangents to the outer and inner circle respectively. If PA = 10cm, find the length of PB up to one place of the decimal.
उत्तर
Given, O is the center of two concentric circles of radii OA = 6 cm and OB = 4 cm.
PA and PB are the two tangents to the outer and inner circles respectively and PA = 10 cm.
Now, tangent drawn from an external point is perpendicular to the radius at the point of contact.
∴∠OAP = ∠OBP = 90°
∴ From right – angled ΔOAP,OP2 = OA2 + PA2
`⇒OP = sqrt(OA^2 + PA^2)`
`⇒ OP = sqrt (6^2 +10^2)`
`⇒ OP = sqrt( 136cm)`.
∴ From right – angled `Δ OAP,OP^2 = OB^2 + PB^2`
`⇒ PB = sqrt(OP^2 -OB^2)`
`⇒ PB = sqrt(136-16)`
`⇒ PB= sqrt(120cm)`
⇒ PB = 109.cm.
∴ The length of PB is 10.9 cm.
APPEARS IN
संबंधित प्रश्न
In the given figure, PA and PB are two tangents to the circle with centre O. If ∠APB = 50° then what is the measure of ∠OAB.

A chord of a circle of radius 14 cm subtends an angle of 120° at the centre. Find the area of the corresponding minor segment of the circle. `[User pi22/7 and sqrt3=1.73]`
The greatest chord of a circle is called its

In the above figure, `square`XLMT is a rectangle. LM = 21 cm, XL = 10.5 cm. Diameter of the smaller semicircle is half the diameter of the larger semicircle. Find the area of non-shaded region.
Given: A circle inscribed in a right angled ΔABC. If ∠ACB = 90° and the radius of the circle is r.
To prove: 2r = a + b – c
In figure, AB is a chord of the circle and AOC is its diameter such that ∠ACB = 50°. If AT is the tangent to the circle at point A, then ∠BAT is equal to ______.
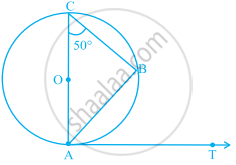
In the given figure, AB is the diameter of the circle. Find the value of ∠ACD.

If AB = 12 cm, BC = 16 cm and AB is perpendicular to BC, then the radius of the circle passing through the points A, B and C is ______.
In the following figure, O is the centre of the circle, ∠BCO = 30°. Find x and y.

Find the length of the arc of a circle which subtends an angle of 60° at the centre of the circle of radius 42 cm.
