Advertisements
Advertisements
प्रश्न
In a process on a system, the initial pressure and volume are equal to the final pressure and volume.
(a) The initial temperature must be equal to the final temperature.
(b) The initial internal energy must be equal to the final internal energy.
(c) The net heat given to the system in the process must be zero.
(d) The net work done by the system in the process must be zero.
उत्तर
(a) The initial temperature must be equal to the final temperature.
(b) The initial internal energy must be equal to the final internal energy.
a) Let initial pressure, volume and temperature be P1, V1 and T1 and final pressure, volume and temperature be P2, V2 and T2. Then,
\[\frac{P_1 V_1}{T_1} = \frac{P_2 V_2}{T_2}\]
\[ \Rightarrow T_1 = T_2...........\left[ P_1 = P_2\text{ and }V_1 = V_2 \right]\]
b) Internal energy is given by ΔU = nCvΔT.
Since ΔT = 0, ΔU = 0.
c) This may not be true because the system may be isothermal and in between, heat may have been given to the system. Also, the system may have done mechanical expansion work and returned back to its original state.
d) Not necessary because an isothermal system may have done work absorbing heat from outside and coming back to the same state losing heat.
APPEARS IN
संबंधित प्रश्न
When we rub our hands they become warm. Have we supplied heat to the hands?
Can work be done by a system without changing its volume?
Figure shows two processes A and B on a system. Let ∆Q1 and ∆Q2 be the heat given to the system in processes A and B respectively. Then ____________ .

Refer to figure. Let ∆U1 and ∆U2 be the changes in internal energy of the system in the process A and B. Then _____________ .

The pressure p and volume V of an ideal gas both increase in a process.
(a) Such a process is not possible.
(b) The work done by the system is positive.
(c) The temperature of the system must increase.
(d) Heat supplied to the gas is equal to the change in internal energy.
Figure shows three paths through which a gas can be taken from the state A to the state B. Calculate the work done by the gas in each of the three paths.
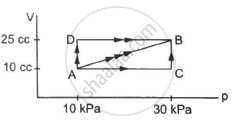
A gas is taken along the path AB as shown in figure. If 70 cal of heat is extracted from the gas in the process, calculate the change in the internal energy of the system.
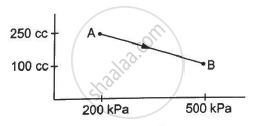
A gas is initially at a pressure of 100 kPa and its volume is 2.0 m3. Its pressure is kept constant and the volume is changed from 2.0 m3 to 2.5 m3. Its Volume is now kept constant and the pressure is increased from 100 kPa to 200 kPa. The gas is brought back to its initial state, the pressure varying linearly with its volume. (a) Whether the heat is supplied to or extracted from the gas in the complete cycle? (b) How much heat was supplied or extracted?
What is the energy associated with the random, disordered motion of the molecules of a system called as?
Define heat.
A cylinder containing one gram molecule of the gas was compressed adiabatically until its temperature rose from 27°C to 97°C. Calculate the work done and heat produced in the gas (𝛾 = 1.5).
derive the relation between the change in internal energy (∆U), work is done (W), and heat (Q).
A thermodynamic system goes from states
(i) P, V to 2P, V (ii) P, V to P, 2V
The work done in the two cases is ____________.
When 1 g of water at 0° C and 1 x 105 N/m2 pressure is converted into ice of volume 1.082 cm3, the external work done will be ____________.
Two cylinders A and B of equal capacity are connected to each other via a stopcock. A contains a gas at standard temperature and pressure. B is completely evacuated. The entire system is thermally insulated. The stopcock is suddenly opened. Answer the following:
What is the change in the temperature of the gas?
A cyclic process ABCA is shown in the V-T diagram. A process on the P-V diagram is ______.
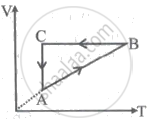
Explain the change in internal energy of a thermodynamic system (the gas) by heating it.
