Advertisements
Advertisements
प्रश्न
In the given figure, AB = DB and Ac = DC.

If ∠ ABD = 58o,
∠ DBC = (2x - 4)o,
∠ ACB = y + 15o and
∠ DCB = 63o ; find the values of x and y.
उत्तर
Given:
In the figure AB = DB, AC = DC, ∠ABD = 58°,
∠DBC = ( 2x - 4 )°, ∠ACB = ( y +15)° and ∠DCB = 63°
We need to find the values of x and y.
In ΔABC and ΔDBC
AB = DB ...[ Given ]
AC= DC ...[ Given ]
BC= BC ...[ common ]
∴ By Side-SIde-Side criterion of congruence, we have,
ΔABC ≅ ΔDBC
The corresponding parts of the congruent triangles are congruent.
∴ ∠ABC= DCB ...[ c. p. c .t ]
⇒ y° + 15° = 63°
⇒ y° = 63° - 15°
⇒ y° = 48°
and ∠ABC =∠DBC ...[ c.p.c.t ]
But, ∠DBC = ( 2x - 4)°
We have ∠ABC + ∠DBC = ∠ABD
⇒ (2x - 4)° + (2x - 4)° = 58°
⇒ 4x - 8°= 58°
⇒ 4x = 58° + 8°
⇒ 4x = 66°
⇒ X = ` 66°/(4)`
⇒ X = 16.5°
Thus the values of x and y are :
x = 16.5° and y = 48°
APPEARS IN
संबंधित प्रश्न
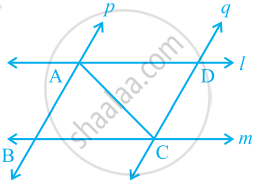
l and m are two parallel lines intersected by another pair of parallel lines p and q (see the given figure). Show that ΔABC ≅ ΔCDA.
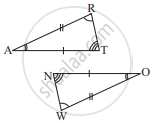
You want to show that ΔART ≅ ΔPEN,
If you have to use SSS criterion, then you need to show
1) AR =
2) RT =
3) AT =

In the figure, the two triangles are congruent.
The corresponding parts are marked. We can write ΔRAT ≅ ?
If perpendiculars from any point within an angle on its arms are congruent, prove that it lies on the bisector of that angle.
Which of the following statements are true (T) and which are false (F):
If any two sides of a right triangle are respectively equal to two sides of other right triangle, then the two triangles are congruent.
In two triangles ABC and ADC, if AB = AD and BC = CD. Are they congruent?
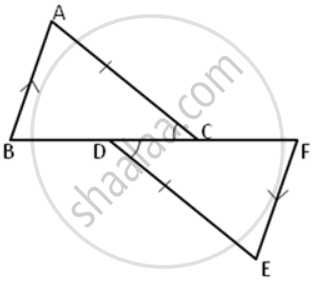
Use the information in the given figure to prove:
- AB = FE
- BD = CF
If the following pair of the triangle is congruent? state the condition of congruency:
In ΔABC and ΔQRP, AB = QR, ∠B = ∠R and ∠C = P.
If AP bisects angle BAC and M is any point on AP, prove that the perpendiculars drawn from M to AB and AC are equal.
From the given diagram, in which ABCD is a parallelogram, ABL is a line segment and E is mid-point of BC.
prove that : AL = 2DC
