Advertisements
Advertisements
प्रश्न
From the given diagram, in which ABCD is a parallelogram, ABL is a line segment and E is mid-point of BC.
Prove that:
(i) ΔDCE ≅ ΔLBE
(ii) AB = BL.
(iii) AL = 2DC
Advertisements
उत्तर
Given: ABCD is a parallelogram in which is the mid-point of BC.
We need to prove that
(i) ΔDCE ≅ ΔLBE
(ii) AB = BL.
(iii) AL = 2DC
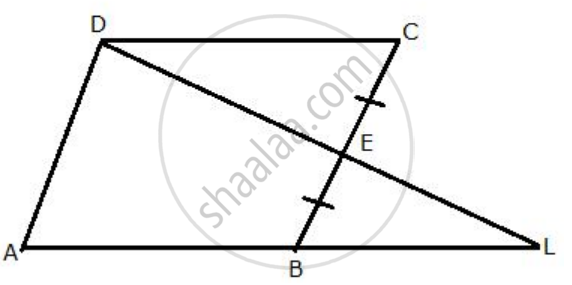
(i) In ΔDCE and ΔLBE
∠DCE = ∠EBL ...[DC || AB, alternate angels]
CE = EB ...[ E is the midpoint of BC]
∠DEC= ∠LEB ...[ vertically opposite angels]
∴ By Angel-SIde-Angel Criterion of congruence, we have,
ΔDCE ≅ ΔLBE
The corresponding parts of the congruent triangles are congruent.
∴ DC= LB ...[ c. p. c .t] ....(1)
(ii) DC= AB ...[ opposite sides of a parallelogram]...(2)
From ( 1 ) and ( 2 ), Ab = BL ...(3)
(iii) Al = AB+ BL ... (4)
From (3) and (4), Al = AB + AB
⇒AL = 2AB
⇒AL = 2DC ...[ From (2) ]
APPEARS IN
संबंधित प्रश्न
AB is a line segment and P is its mid-point. D and E are points on the same side of AB such that ∠BAD = ∠ABE and ∠EPA = ∠DPB (See the given figure). Show that
- ΔDAP ≅ ΔEBP
- AD = BE
You want to show that ΔART ≅ ΔPEN,
If it is given that ∠T = ∠N and you are to use SAS criterion, you need to have
1) RT = and
2) PN =
If perpendiculars from any point within an angle on its arms are congruent, prove that it lies on the bisector of that angle.
In the given figure, prove that:
CD + DA + AB + BC > 2AC
If the following pair of the triangle is congruent? state the condition of congruency :
In Δ ABC and Δ DEF, AB = DE, BC = EF and ∠ B = ∠ E.
Use the information in the given figure to prove:
- AB = FE
- BD = CF
The given figure shows a circle with center O. P is mid-point of chord AB.
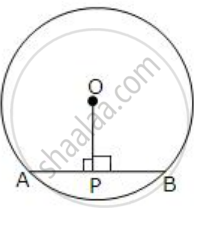
Show that OP is perpendicular to AB.
In the following figure, AB = AC and AD is perpendicular to BC. BE bisects angle B and EF is perpendicular to AB.
Prove that : ED = EF
In the following figures, the sides AB and BC and the median AD of triangle ABC are equal to the sides PQ and QR and median PS of the triangle PQR.
Prove that ΔABC and ΔPQR are congruent.
 |
 |
In the parallelogram ABCD, the angles A and C are obtuse. Points X and Y are taken on the diagonal BD such that the angles XAD and YCB are right angles.
Prove that: XA = YC.
