Advertisements
Advertisements
प्रश्न
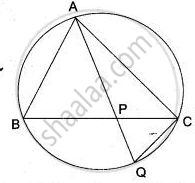
In the given Figure. P is any point on the chord BC of a circle such that AB = AP. Prove that CP = CQ.
उत्तर
We have to prove that CP = CQ i.e., Δ CPQ is an isosceles triangle. for this it is sufficient to prove that ∠ CPQ = ∠ CQP.
In Δ ABP, we have
AB = AP
⇒ ∠ APB = ∠ ABP
⇒ ∠ CPQ = ∠ ABP ...(i)( ∵ ∠APB and ∠ CPQ are vertically opposite angles ∴ ∠APB = ∠ CPQ )
Now consider arc AC. Clearly, it subtends ∠ABC and ∠AQC at points B and Q.
∴ ∠ABC = ∠AQC ...( ∵ Angles in the same segment)
⇒ ∠ABP = ∠PQC ...( ∵∠ ABC = ∠ ABP and ∠AQC = ∠PQC )
⇒ ∠ABP = ∠CQP ....(ii)( ∵ ∠PQC = ∠CQP )
From (i) and (ii), we get
∠ CPQ = ∠CQP
⇒ CQ = CP
Hence proved.
APPEARS IN
संबंधित प्रश्न
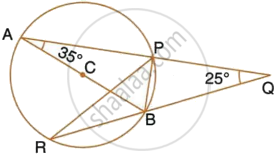
AB is a diameter of the circle APBR as shown in the figure. APQ and RBQ are straight lines. Find : ∠PRB
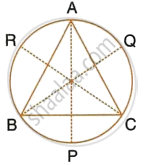
A triangle ABC is inscribed in a circle. The bisectors of angles BAC, ABC and ACB meet the circumcircle of the triangle at points P, Q and R respectively. Prove that:
- ∠ABC = 2∠APQ,
- ∠ACB = 2∠APR,
- `∠QPR = 90^circ - 1/2 ∠BAC`.
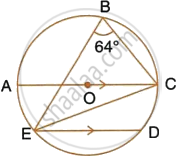
In the given figure, AOC is a diameter and AC is parallel to ED. If ∠CBE = 64°, calculate ∠DEC.
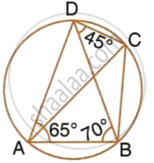
In the given figure, ∠BAD = 65°, ∠ABD = 70° and ∠BDC = 45°. Find:
- ∠BCD
- ∠ACB
Hence, show that AC is a diameter.
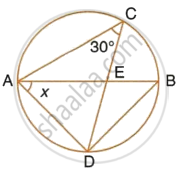
In the given circle with diameter AB, find the value of x.
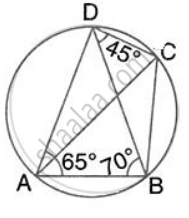
In cyclic quadrilateral ABCD; AD = BC, ∠BAC = 30° and ∠CBD = 70°; find:
- ∠BCD
- ∠BCA
- ∠ABC
- ∠ADC
In the figure, given below, AD = BC, ∠BAC = 30° and ∠CBD = 70°.
Find:
- ∠BCD
- ∠BCA
- ∠ABC
- ∠ADB

In the given figure, ∠BAD = 65°, ∠ABD = 70° and ∠BDC = 45°. Find: ∠ ACB.
Hence, show that AC is a diameter.
If I is the incentre of triangle ABC and AI when produced meets the cicrumcircle of triangle ABC in points D . if ∠BAC = 66° and ∠ABC = 80°. Calculate : ∠IBC
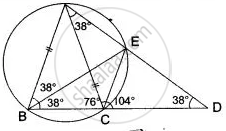
In the figure, AB = AC = CD, ∠ADC = 38°. Calculate: (i) ∠ ABC, (ii) ∠ BEC.