Advertisements
Advertisements
प्रश्न
median of a triangle divides it into two triangles of equal areas. Verify this result for ΔABC whose vertices are A (4, - 6), B (3, - 2) and C (5, 2).
उत्तर
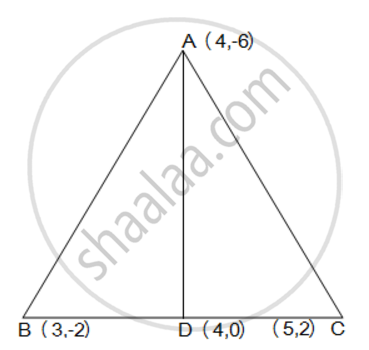
Let the vertices of the triangle be A (4, -6), B (3, -2), and C (5, 2).
Let D be the mid-point of side BC of ΔABC. Therefore, AD is the median in ΔABC.
Coordinates of point `D = ((3+5)/2, (-2+2)/2) = (4,0)`
Area of a triangle =`1/2 {x_1 (y_2 - y_3) + x_2 (y_3 - y_1) + x_3 (y_1 - y_2)}`
Area of ΔABD = ` 1/2 [(4) {(-2) - (0)} + 3{(0) - (-6)} + (4) {(-6) - (-2)}]`
= 1/2 (-8+18-16)
= -3 square units
However, area cannot be negative. Therefore, area of ΔABD is 3 square units.
Area of ΔABD = `1/2 [(4) {0 - (2)} + 4{(2) - (-6)} + (5) {(-6) - (0)}]`
= 1/2 (-8+32-30)
= -3 square units
However, area cannot be negative. Therefore, area of ΔABD is 3 square units.
The area of both sides is same. Thus, median AD has divided ΔABC in two triangles of equal areas
APPEARS IN
संबंधित प्रश्न
Determine the ratio in which the line 2x + y – 4 = 0 divides the line segment joining the points A(2, – 2) and B(3, 7).
Find the area of a triangle with vertices at the point given in the following:
(−2, −3), (3, 2), (−1, −8)
Find values of k if area of triangle is 4 square units and vertices are (−2, 0), (0, 4), (0, k)
Find equation of line joining (3, 1) and (9, 3) using determinant.
A(7, -3), B(5,3) and C(3,-1) are the vertices of a ΔABC and AD is its median. Prove that the median AD divides ΔABC into two triangles of equal areas.
A(6,1) , B(8,2) and C(9,4) are the vertices of a parallelogram ABCD. If E is the midpoint of DC, find the area of ΔADE
Find the value of p for which the points (−5, 1), (1, p) and (4, −2) are collinear.
Using integration, find the area of triangle ABC, whose vertices are A(2, 5), B(4, 7) and C(6, 2).
The table given below contains some measures of the right angled triangle. Find the unknown values.
| Base | Height | Area |
| ? | 12 m | 24 sq.m |
Area of a right-angled triangle is 30 cm2. If its smallest side is 5 cm, then its hypotenuse is ______.
