Advertisements
Advertisements
प्रश्न
Find equation of line joining (3, 1) and (9, 3) using determinant.
उत्तर
Let P(x, y) be the point on the line joining the points A(3, 1) and B(9, 3). Then the points A, P and B are collinear.
∴ Area (∆ APB) = 0
`=> 1/2 abs ((3,1,1),(9,3,1),(x,y,1)) = 0`
`=> abs ((3,1,1),(9,3,1),(x,y,1)) - 0`
⇒ x (1 - 3) - y (3 - 9) + 1 (9 - 9) = 0
⇒ -2x + 6y = 0
⇒ x - 3y = 0
Hence, x - 3y = 0 is the required line.
APPEARS IN
संबंधित प्रश्न
Find the values of k so that the area of the triangle with vertices (k + 1, 1), (4, -3) and (7, -k) is 6 sq. units.
In Fig. 6, ABC is a triangle coordinates of whose vertex A are (0, −1). D and E respectively are the mid-points of the sides AB and AC and their coordinates are (1, 0) and (0, 1) respectively. If F is the mid-point of BC, find the areas of ∆ABC and ∆DEF.

Prove that the area of a triangle with vertices (t, t −2), (t + 2, t + 2) and (t + 3, t) is independent of t.
Find the area of the triangle ABC with A(1, −4) and mid-points of sides through A being (2, −1) and (0, −1).
Find the area of the triangle PQR with Q(3,2) and the mid-points of the sides through Q being (2,−1) and (1,2).
Show that points A (a, b + c), B (b, c + a), C (c, a + b) are collinear.
Find values of k if area of triangle is 4 square units and vertices are (k, 0), (4, 0), (0, 2)
If area of triangle is 35 square units with vertices (2, −6), (5, 4), and (k, 4), then k is ______.
Find the area of the following triangle:

ΔABC is right angled at A (see the given figure). AD is perpendicular to BC. If AB = 5 cm, BC = 13 cm and AC = 12 cm, Find the area of ΔABC. Also find the length of AD.

The area of a triangle is 5. Two of its vertices are (2, 1) and (3, −2). The third vertex lies on y = x + 3. Find the third vertex.
Find the area of a triangle whose sides are respectively 150 cm, 120 cm and 200 cm ?
prove that the points A (7, 10), B(-2, 5) and C(3, -4) are the vertices of an isosceles right triangle.
Show that the points A(-5,6), B(3,0) and C(9,8) are the vertices of an isosceles right-angled triangle. Calculate its area.
Find the area of ΔABC whose vertices are:
A( 3,8) , B(-4,2) and C( 5, -1)
A(6,1) , B(8,2) and C(9,4) are the vertices of a parallelogram ABCD. If E is the midpoint of DC, find the area of ΔADE
Show that the following points are collinear:
A(8,1), B(3, -4) and C(2, -5)
Find the value of x for which the points (x, −1), (2, 1) and (4, 5) are collinear ?
Find the value(s) of k so that the quadratic equation x2 − 4kx + k = 0 has equal roots.
A field is in the shape of a right angled triangle whose base is 25 m and height 20 m. Find the cost of levelling the field at the rate of ₹ 45 per sq.m2
If A, B, C are the angles of a triangle, then ∆ = `|(sin^2"A", cot"A", 1),(sin^2"B", cot"B", 1),(sin^2"C", cot"C", 1)|` = ______.
Show that the ∆ABC is an isosceles triangle if the determinant
Δ = `[(1, 1, 1),(1 + cos"A", 1 + cos"B", 1 + cos"C"),(cos^2"A" + cos"A", cos^2"B" + cos"B", cos^2"C" + cos"C")]` = 0
The value of the determinant `abs((1,"x","x"^3),(1,"y","y"^3),(1,"z","z"^3))` is ____________.
Points A(3, 1), B(12, –2) and C(0, 2) cannot be the vertices of a triangle.
A(6, 1), B(8, 2) and C(9, 4) are three vertices of a parallelogram ABCD. If E is the midpoint of DC, find the area of ∆ADE.
Find the coordinates of the point Q on the x-axis which lies on the perpendicular bisector of the line segment joining the points A(–5, –2) and B(4, –2). Name the type of triangle formed by the points Q, A and B.
If `D((-1)/2, 5/2), E(7, 3)` and `F(7/2, 7/2)` are the midpoints of sides of ∆ABC, find the area of the ∆ABC.
The area of a triangle with base 4 cm and height 6 cm is 24 cm2.
The area of ∆ABC is 8 cm2 in which AB = AC = 4 cm and ∠A = 90º.
A rectangular plot is given for constructing a house, having a measurement of 40 m long and 15 m in the front. According to the laws, a minimum of 3 m, wide space should be left in the front and back each and 2 m wide space on each of other sides. Find the largest area where house can be constructed.
Ratio of areas of ∆MNO, ∆MOP and ∆MPQ in the given figure is ______.

Area of a right-angled triangle is 30 cm2. If its smallest side is 5 cm, then its hypotenuse is ______.
Observe all the four triangles FAB, EAB, DAB and CAB as shown in the given figure.

- All triangles have the same base and the same altitude.
- All triangles are congruent.
- All triangles are equal in area.
- All triangles may not have the same perimeter.
Ratio of the area of ∆WXY to the area of ∆WZY is 3:4 in the given figure. If the area of ∆WXZ is 56 cm2 and WY = 8 cm, find the lengths of XY and YZ.

Area of a triangle PQR right-angled at Q is 60 cm2 in the figure. If the smallest side is 8 cm long, find the length of the other two sides.

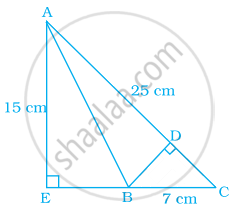
In the given figure, triangle AEC is right-angled at E, B is a point on EC, BD is the altitude of triangle ABC, AC = 25 cm, BC = 7 cm and AE = 15 cm. Find the area of triangle ABC and the length of DB.