Advertisements
Advertisements
प्रश्न
Sketch the graph of f, then identify the values of x0 for which `lim_(x -> x_0)` f(x) exists.
f(x) = `{{:(sin x",", x < 0),(1 - cos x",", 0 ≤ x ≤ pi),(cos x",", x > pi):}`
उत्तर
f(x) = `{{:(sin x",", x < 0),(1 - cos x",", 0 ≤ x ≤ pi),(cos x",", x > pi):}`
From the figure when x = π, y = f(π) = 2.
The function is not defined at x = π since sin x lies in the interval [– 1, 1]
∴ The given function has limits at all points except at x = π
| x | `- pi/2` | 0 | `pi/2` | `pi` | `(3pi)/2` | 2 |
| f(x) | `sin (- pi/2)` | 1 – cos 0 | `1 - cos pi/2` | 1 – cos π | `cos((3pi)/2)` | cos 2π |
| f(x) | – 1 | 0 | 1 | 1 – (– 1) = 2 | 0 | 1 |
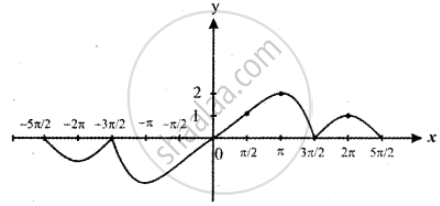
(π, 2) point is not possible since the range of the curve is [– 1, 1] .
Except x0 = π, the curve has limits.
APPEARS IN
संबंधित प्रश्न
Evaluate the following limit:
`lim_(y -> -3) [(y^5 + 243)/(y^3 + 27)]`
Evaluate the following limit :
`lim_(x -> 1) [(x + x^3 + x^5 + ... + x^(2"n" - 1) - "n")/(x - 1)]`
Evaluate the following :
`lim_(x -> 0)[x/(|x| + x^2)]`
In problems 1 – 6, using the table estimate the value of the limit
`lim_(x -> 0) sin x/x`
| x | – 0.1 | – 0.01 | – 0.001 | 0.001 | 0.01 | 0.1 |
| f(x) | 0.99833 | 0.99998 | 0.99999 | 0.99999 | 0.99998 | 0.99833 |
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 1) f(x)` where `f(x) = {{:(x^2 + 2",", x ≠ 1),(1",", x = 1):}`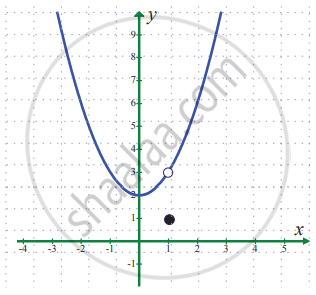
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 3) 1/(x - 3)`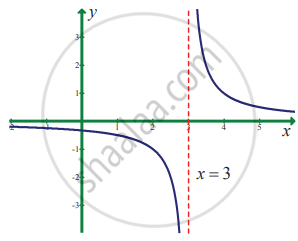
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 1) sin pi x`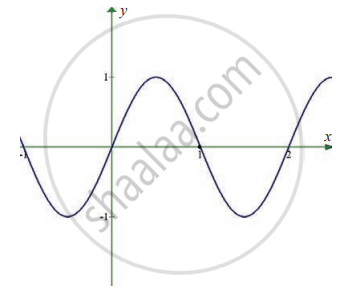
Evaluate the following limits:
`lim_(x -> 2) (x^4 - 16)/(x - 2)`
Evaluate the following limits:
`lim_(x -> 0) (sqrt(x^2 + 1) - 1)/(sqrt(x^2 + 16) - 4)`
Evaluate the following limits:
`lim_(x -> 5) (sqrt(x - 1) - 2)/(x - 5)`
Evaluate the following limits:
`lim_(x -> "a") (sqrt(x - "b") - sqrt("a" - "b"))/(x^2 - "a"^2) ("a" > "b")`
Evaluate the following limits:
`lim_(x -> 3) (x^2 - 9)/(x^2(x^2 - 6x + 9))`
Evaluate the following limits:
`lim_(x -> oo) (1 + x - 3x^3)/(1 + x^2 +3x^3)`
Show that `lim_("n" -> oo) (1^2 + 2^2 + ... + (3"n")^2)/((1 + 2 + ... + 5"n")(2"n" + 3)) = 9/25`
Evaluate the following limits:
`lim_(x-> 0) (1 - cos x)/x^2`
Evaluate the following limits:
`lim_(x -> 0) (1 - cos^2x)/(x sin2x)`
Evaluate the following limits:
`lim_(x -> oo) ((x^2 - 2x + 1)/(x^2 -4x + 2))^x`
Evaluate the following limits:
`lim_(x -> 0) ("e"^x - "e"^(-x))/sinx`
`lim_(x -> 0) ((2 + x)^5 - 2)/((2 + x)^3 - 2)` = ______.
`lim_(x→-1) (x^3 - 2x - 1)/(x^5 - 2x - 1)` = ______.
