Advertisements
Advertisements
प्रश्न
Solve each of the following system of equations in R.
2x − 7 > 5 − x, 11 − 5x ≤ 1
उत्तर
Given as
2x – 7 > 5 – x and 11 – 5x ≤ 1
Now, let us consider the first inequality.
2x – 7 > 5 – x
2x – 7 + 7 > 5 – x + 7
2x > 12 – x
2x + x > 12 – x + x
3x > 12
Dividing both the sides by 3 we get,
`(3x)/3 > 12/3`
x > 4
∴ x ∈ (4, ∞) ...(1)
Then, let us consider the second inequality.
11 – 5x ≤ 1
11 – 5x – 11 ≤ 1 – 11
– 5x ≤ – 10
Dividing both the sides by 5 we get,
`(– 5x)/5 ≤ (–10)/5`
–x ≤ –2
x ≥ 2
∴ x ∈ (2, ∞) ...(2)
From (1) and (2) we get
x ∈ (4, ∞) ∩ (2, ∞)
x ∈ (4, ∞)
Hence, the solution of the given system of inequations is (4, ∞).
APPEARS IN
संबंधित प्रश्न
Solve: −4x > 30, when x ∈ N
Solve: 4x − 2 < 8, when x ∈ N
3x + 9 ≥ −x + 19
\[2\left( 3 - x \right) \geq \frac{x}{5} + 4\]
\[\frac{5 - 2x}{3} < \frac{x}{6} - 5\]
\[x - 2 \leq \frac{5x + 8}{3}\]
\[\frac{4x + 3}{2x - 5} < 6\]
\[\frac{5x + 8}{4 - x} < 2\]
\[\frac{x - 1}{x + 3} > 2\]
Solve each of the following system of equations in R.
1. x + 3 > 0, 2x < 14
Solve each of the following system of equations in R.
2x − 3 < 7, 2x > −4
Solve each of the following system of equations in R.
2x + 5 ≤ 0, x − 3 ≤ 0
Solve each of the following system of equations in R.
3x − 1 ≥ 5, x + 2 > −1
Solve each of the following system of equations in R.
4x − 1 ≤ 0, 3 − 4x < 0
Solve \[\left| x - 1 \right| + \left| x - 2 \right| + \left| x - 3 \right| \geq 6\]
Solve \[1 \leq \left| x - 2 \right| \leq 3\]
Mark the correct alternative in each of the following:
Given that x, y and b are real numbers and x\[<\]y, b\[>\]0, then
Mark the correct alternative in each of the following:
The linear inequality representing the solution set given in 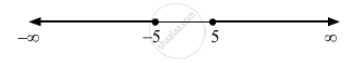
Mark the correct alternative in each of the following:
The solution set of the inequation \[\left| x + 2 \right|\]\[\leq\]5 is
Solve the inequality, 3x – 5 < x + 7, when x is a natural number.
Solve the inequality, 3x – 5 < x + 7, when x is a whole number.
Solve `(x - 2)/(x + 5) > 2`.
Solve 1 ≤ |x – 2| ≤ 3.
The cost and revenue functions of a product are given by C(x) = 20x + 4000 and R(x) = 60x + 2000, respectively, where x is the number of items produced and sold. How many items must be sold to realise some profit?
Solve for x, |x + 1| + |x| > 3.
Solve the following system of inequalities:
`x/(2x + 1) ≥ 1/4, (6x)/(4x - 1) < 1/2`
If |x + 3| ≥ 10, then ______.
If a < b and c < 0, then `a/c` ______ `b/c`.
If |x − 1| ≤ 2, then –1 ______ x ______ 3
Solve for x, the inequality given below.
`(|x - 2| - 1)/(|x - 2| - 2) ≤ 0`
If x < 5, then ______.
Given that x, y and b are real numbers and x < y, b < 0, then ______.
If – 4x ≥ 12, then x ______ – 3.
