Advertisements
Advertisements
प्रश्न
The angle of elevation of a cloud from a point 60m above a lake is 30° and the angle of depression of its reflection in the lake is 60°. Find the height of the cloud.
उत्तर
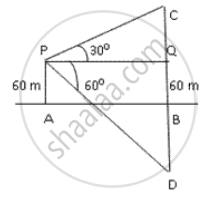
Let C be the cloud and D be its reflection . Let the height of the cloud is h metres .
BC = BD = h
BQ = AP = 60m
∴ CQ = h - 60 and DQ = h + 60
In ΔCQP,
`"PQ"/"CQ" = cot30^circ`
⇒ `"PQ"/("h" - 60) = sqrt(3)`
⇒ `"PQ" = sqrt(3)("h" - 60)"` ....(i)
In ΔDQP,
`"PQ"/"DQ" = cot60^circ`
⇒ `"PQ"/("h" + 60) = 1/sqrt(3)`
⇒ `"PQ" = 1/sqrt(3)("h" + 60)` ..(ii)
From (i) and (ii),
⇒ `sqrt(3)("h" - 60) = 1/sqrt(3)("h" + 60)`
⇒ 3h - 180 = h + 60
⇒ 2h = 240
⇒ h = 120
Thus , the height of the cloud is 120 m.
APPEARS IN
संबंधित प्रश्न
A bus covers a distance of 240 km at a uniform speed. Due to heavy rain, its speed gets reduced by 10 km/h and as such it takes two hrs longer to cover the total distance. Assuming the uniform speed to be ‘x’ km/h, form an equation and solve it to evaluate ‘x’.
In the figure given, from the top of a building AB = 60 m high, the angles of depression of the top and bottom of a vertical lamp post CD are observed to 30o and 60o respectively. Find:
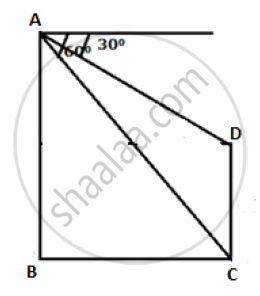
1) The horizontal distance between AB and CD.
2) The height of the lamp post.
The angle of elevation of the top of a vertical cliff from a point 30 m away from the foot of the cliff is 60°. Find the height of the cliff.
The top of a ladder reaches a pcint on the wall 5 m above the ground. If the foot of the ladder makes an angle of 30° with the ground, find the length of the ladder.
The horizontal distance between two trees of different heights is 100m. The angle of depression of the top of the first tree when seen from the top of the second tree is 45°. If the height of the second tree is 150m, find the height of the first tree.
Two boats approaching a light house in mid sea from opposite directions observe the angle of elevation of the top of the light house as 30° and 45° respectively. If the distance between the two boats is 150m, find the height of the light house.
A man standing on a cliff observes a ship at an angle of depression of the ship is 30°, approaching the shore just beneath him. Three minutes later, the angle of depression of the ship is 60°. How soon will it reach the shore?
From an aeroplane vertically above a straight horizontal road, the angles of depression of two consecutive milestone on opposite sides of the aeroplane are observed to be α, and β. Show that the height in miles of aeroplane above the road is `(tanα tanβ)/(tanα + tanβ)`.
In figures, find the length CF.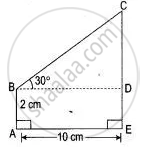
A man on the top of vertical observation tower observes a car moving at a uniform speed coming directly towards it. If it takes 12 minutes for the angle of depression to change from 30° to 45°, how soon after this will the car reach the observation tower? (Give your answer correct to nearest seconds).
