Advertisements
Advertisements
प्रश्न
The curves y = 4x2 + 2x – 8 and y = x3 – x + 13 touch each other at the point ______.
उत्तर
The curves y = 4x2 + 2x – 8 and y = x3 – x + 13 touch each other at the point `(- 1/3, (-74)/9)`.
Explanation:
We have y = 4x2 + 2x – 8 .....(i)
And y = x3 – x + 13 .....(ii)
Differentiating eq. (i) w.r.t. x, we have
`"dy"/'dx"` = 8x + 2
⇒ m1 = 8x + 2 .....[m is the slope of curve (i)]
Differentiating eq. (ii) w.r.t. x, we get
`"dy"/"dx"` = 3x2 – 1
⇒ m2 = 3x2 – 1 ......[m2 is the slope of curve (ii)]
If the two curves touch each other, then m1 = m2
∴ 8x + 2 = 3x2 – 1
⇒ 3x2 – 8x – 3 = 0
⇒ 3x2 – 9x + x – 3 = 0
⇒ 3x(x – 3) + 1(x – 3) = 0
⇒ (x – 3)(3x + 1) = 0
∴ x = 3, `(-1)/3`
Putting x = 3 in equation (i), we get
y = 4(3)2 + 2(3) – 8
= 36 + 6 – 8
= 34
So, the required point is (3, 34)
Now for x = `- 1/3`
y = `4((-1)/3)^2 + 2((-1)/3) - 8`
= `4 xx 1/9 - 2/3 - 8`
= `4/9 - 2/3 - 8`
= `(4 - 6 - 72)/9`
= `(-74)/9`
∴ Other required point is `(- 1/3, (-74)/9)`.
APPEARS IN
संबंधित प्रश्न
Find the absolute maximum value and the absolute minimum value of the following function in the given interval:
f (x) = sin x + cos x , x ∈ [0, π]
A square piece of tin of side 18 cm is to made into a box without a top by cutting a square from each corner and folding up the flaps to form the box. What should be the side of the square to be cut off so that the volume of the box is the maximum possible?
A given quantity of metal is to be cast into a half cylinder with a rectangular base and semicircular ends. Show that in order that the total surface area may be minimum the ratio of the length of the cylinder to the diameter of its semi-circular ends is \[\pi : (\pi + 2)\].
Find the point on the straight line 2x+3y = 6, which is closest to the origin.
Divide the number 20 into two parts such that sum of their squares is minimum.
The perimeter of a triangle is 10 cm. If one of the side is 4 cm. What are the other two sides of the triangle for its maximum area?
Solve the following : Show that the height of a right circular cylinder of greatest volume that can be inscribed in a right circular cone is one-third of that of the cone.
Solve the following:
A wire of length l is cut into two parts. One part is bent into a circle and the other into a square. Show that the sum of the areas of the circle and the square is the least, if the radius of the circle is half of the side of the square.
Determine the maximum and minimum value of the following function.
f(x) = x log x
Divide the number 20 into two parts such that their product is maximum.
If f(x) = x.log.x then its maximum value is ______.
If x + y = 3 show that the maximum value of x2y is 4.
A rod of 108 m long is bent to form a rectangle. Find it’s dimensions when it’s area is maximum.
Twenty meters of wire is available for fencing off a flowerbed in the form of a circular sector. Then the maximum area (in sq. m) of the flower-bed, is ______
The maximum and minimum values for the function f(x) = 4x3 - 6x2 on [-1, 2] are ______
Show that the function f(x) = 4x3 – 18x2 + 27x – 7 has neither maxima nor minima.
If the sum of the lengths of the hypotenuse and a side of a right-angled triangle is given, show that the area of the triangle is maximum when the angle between them is `pi/3`
The smallest value of the polynomial x3 – 18x2 + 96x in [0, 9] is ______.
The maximum value of `["x"("x" − 1) + 1]^(1/3)`, 0 ≤ x ≤ 1 is:
If y `= "ax - b"/(("x" - 1)("x" - 4))` has a turning point P(2, -1), then find the value of a and b respectively.
The function f(x) = x5 - 5x4 + 5x3 - 1 has ____________.
The function `f(x) = x^3 - 6x^2 + 9x + 25` has
Read the following passage and answer the questions given below.
|
|
- Is the function differentiable in the interval (0, 12)? Justify your answer.
- If 6 is the critical point of the function, then find the value of the constant m.
- Find the intervals in which the function is strictly increasing/strictly decreasing.
OR
Find the points of local maximum/local minimum, if any, in the interval (0, 12) as well as the points of absolute maximum/absolute minimum in the interval [0, 12]. Also, find the corresponding local maximum/local minimum and the absolute ‘maximum/absolute minimum values of the function.
A wire of length 22 m is to be cut into two pieces. One of the pieces is to be made into a square and the other into an equilateral triangle. Then, the length of the side of the equilateral triangle, so that the combined area of the square and the equilateral triangle is minimum, is ______.
The range of a ∈ R for which the function f(x) = `(4a - 3)(x + log_e5) + 2(a - 7)cot(x/2)sin^2(x/2), x ≠ 2nπ, n∈N` has critical points, is ______.
If p(x) be a polynomial of degree three that has a local maximum value 8 at x = 1 and a local minimum value 4 at x = 2; then p(0) is equal to ______.
A running track of 440 m is to be laid out enclosing a football field. The football field is in the shape of a rectangle with a semi-circle at each end. If the area of the rectangular portion is to be maximum,then find the length of its sides. Also calculate the area of the football field.
Sumit has bought a closed cylindrical dustbin. The radius of the dustbin is ‘r' cm and height is 'h’ cm. It has a volume of 20π cm3.
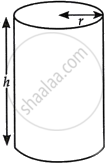
- Express ‘h’ in terms of ‘r’, using the given volume.
- Prove that the total surface area of the dustbin is `2πr^2 + (40π)/r`
- Sumit wants to paint the dustbin. The cost of painting the base and top of the dustbin is ₹ 2 per cm2 and the cost of painting the curved side is ₹ 25 per cm2. Find the total cost in terms of ‘r’, for painting the outer surface of the dustbin including the base and top.
- Calculate the minimum cost for painting the dustbin.

