Advertisements
Advertisements
प्रश्न
The position, velocity and acceleration of a particle executing simple harmonic motion are found to have magnitude 2 cm, 1 m s−1 and 10 m s−2 at a certain instant. Find the amplitude and the time period of the motion.
उत्तर
It is given that:
Position of the particle, x = 2 cm = 0.02 m
Velocity of the particle, v = 1 ms−1.
Acceleration of the particle, a = 10 ms−2.
Let
\[\omega\] be the angular frequency of the particle.
The acceleration of the particle is given by,
a = ω2x
\[\Rightarrow \omega = \sqrt{\frac{a}{x}} = \sqrt{\frac{10}{0 . 02}}\]
\[ = \sqrt{500} = 10\sqrt{5} Hz\]
\[\text { Time period of the motion is given as, } \]
\[ T = \frac{2\pi}{\omega} = \frac{2\pi}{10\sqrt{5}}\]
\[ = \frac{2 \times 3 . 14}{10 \times 2 . 236}\]
\[ = 0 . 28 s\]
Now, the amplitude A is calculated as,
\[v = \omega\sqrt{A^2 - x^2}\]
\[ \Rightarrow v^2 = \omega^2 \left( A^2 - x^2 \right)\]
\[ 1 = 500\left( A^2 - 0 . 0004 \right)\]
\[ \Rightarrow A = 0 . 0489 = 0 . 049 m\]
\[ \Rightarrow A = 4 . 9 \text { cm }\]
APPEARS IN
संबंधित प्रश्न
A seconds pendulum is suspended in an elevator moving with constant speed in downward direction. The periodic time (T) of that pendulum is _______.
A copper metal cube has each side of length 1 m. The bottom edge of the cube is fixed and tangential force 4.2x108 N is applied to a top surface. Calculate the lateral displacement of the top surface if modulus of rigidity of copper is 14x1010 N/m2.
The piston in the cylinder head of a locomotive has a stroke (twice the amplitude) of 1.0 m. If the piston moves with simple harmonic motion with an angular frequency of 200 rad/min, what is its maximum speed?
The length of the second’s pendulum in a clock is increased to 4 times its initial length. Calculate the number of oscillations completed by the new pendulum in one minute.
A person goes to bed at sharp 10.00 pm every day. Is it an example of periodic motion? If yes, what is the time period? If no, why?
The total mechanical energy of a spring-mass system in simple harmonic motion is \[E = \frac{1}{2}m \omega^2 A^2 .\] Suppose the oscillating particle is replaced by another particle of double the mass while the amplitude A remains the same. The new mechanical energy will
A particle moves in a circular path with a uniform speed. Its motion is
Consider a simple harmonic motion of time period T. Calculate the time taken for the displacement to change value from half the amplitude to the amplitude.
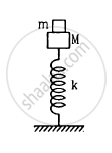
A small block of mass m is kept on a bigger block of mass M which is attached to a vertical spring of spring constant k as shown in the figure. The system oscillates vertically. (a) Find the resultant force on the smaller block when it is displaced through a distance x above its equilibrium position. (b) Find the normal force on the smaller block at this position. When is this force smallest in magnitude? (c) What can be the maximum amplitude with which the two blocks may oscillate together?
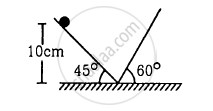
Find the time period of the motion of the particle shown in figure . Neglect the small effect of the bend near the bottom.
A uniform plate of mass M stays horizontally and symmetrically on two wheels rotating in opposite direction in Figure . The separation between the wheels is L. The friction coefficient between each wheel and the plate is μ. Find the time period of oscillation of the plate if it is slightly displaced along its length and released.

The ear-ring of a lady shown in figure has a 3 cm long light suspension wire. (a) Find the time period of small oscillations if the lady is standing on the ground. (b) The lady now sits in a merry-go-round moving at 4 m/s1 in a circle of radius 2 m. Find the time period of small oscillations of the ear-ring.

A uniform disc of radius r is to be suspended through a small hole made in the disc. Find the minimum possible time period of the disc for small oscillations. What should be the distance of the hole from the centre for it to have minimum time period?
The period of oscillation of a body of mass m1 suspended from a light spring is T. When a body of mass m2 is tied to the first body and the system is made to oscillate, the period is 2T. Compare the masses m1 and m2
A simple pendulum is inside a spacecraft. What will be its periodic time?
When two displacements represented by y1 = a sin(ωt) and y2 = b cos(ωt) are superimposed the motion is ______.
What are the two basic characteristics of a simple harmonic motion?
A particle performs simple harmonic motion with a period of 2 seconds. The time taken by the particle to cover a displacement equal to half of its amplitude from the mean position is `1/a` s. The value of 'a' to the nearest integer is ______.
