Advertisements
Advertisements
प्रश्न
Two circle with radii r1 and r2 touch each other externally. Let r be the radius of a circle which touches these two circle as well as a common tangent to the two circles, Prove that: `1/sqrtr + 1/sqrtr_1 + 1/sqrtr_2`.
उत्तर
From the adjoining figure,
PQ = SY = `sqrt( "XY"^2 - "XS"^2)`
= `sqrt((r_1 + r_2)^2 - (r_1 - r_2)^2)`
= `sqrt(4r_1r_2)`
= `sqrt(r_1r_2)`

Similarly, PR = `2 sqrt(rr_1)` and RQ = `2 sqrt(rr_2)`
Now, PQ = PR + RQ
`2 sqrt(r_1r_2) = 2 sqrt(rr_1) = 2 sqrt(rr_2) `
⇒ `sqrt(r_1r_2) = sqrt(rr_1) = sqrt(rr_2) `
Dividing by `sqrt(rr_1r_2)` on both sides,
⇒ `1/sqrtr + 1/sqrtr_1 + 1/sqrtr_2`.
Hence proved.
APPEARS IN
संबंधित प्रश्न
In the given figure, BDC is a tangent to the given circle at point D such that BD = 30 cm and CD = 7 cm. The other tangents BE and CF are drawn respectively from B and C to the circle and meet when produced at A making BAC a right angle triangle. Calculate (ii) radius of the circle.

In fig., AB and DC are two chords of a circle with centre O. these chords when produced meet at P. if PB = Bern, BA = 7cm and PO = 14.5cm, find the radius of the circle.

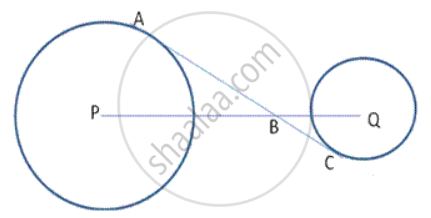
In the following fig. , AC is a transversal common tangent to tvvo circles with centres P and Q and of radii 6cm and 3cm respectively. Given that AB = 8cm, calculate PQ.
Construct a tangent to a circle with centre O and radius 3.5 cm at a point P on it.
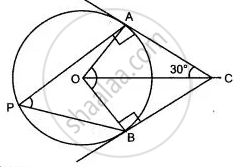
In the given figure, O is the centre of the circle. Tangents at A and B meet at C. If ∠ACO = 30°,
find: (i) ∠ BCO (ii) ∠ AOB (iii) ∠ APB
In the given figure, diameter AB and chord CD of a circle meet at P. PT is a tangent to the circle at T. CD = 7.8 cm, PD = 5 cm, PB = 4 cm. Find:
- AB.
- the length of tangent PT.

The distance between two parallel tangents of a circle of radius 4 cm is ______
The tangents drawn at the extremities of the diameter of a circle are ______.
In the figure, PQ and PR are tangents to a circle with centre A. If ∠QPA=27°, then ∠QAR equals to ______

If two tangents TL and TM are drawn to a circle with centre C such that ∠LTM = 70°, then find ∠MCT.

