Advertisements
Advertisements
Question
A point D is on the side BC of an equilateral triangle ABC such that\[DC = \frac{1}{4}BC\]. Prove that AD2 = 13 CD2.
Solution
We are given ABC is an equilateral triangle with `CD = 1/2 BC`
We have to prove `AD^2 = 13DC^2`
Draw `AE ⊥ BC`
In Δ AEB and ΔAED we have `AB =AC`
`∠ AEB = ∠ AEC = 90^o `
`AE = AE`
So by right side criterion of similarity we have

Thus we have
`DC =1/4BC and BE =EC = 1/2 BC`
Since `∠ C= 60^o` therefore
`AD^2 =AC^2+DC^2 - 2DC xxEC`
`AD^2 = AC^2(1/4BC)^2-2xx1/4BCxx1/2BC`
`AS^2=AC^2+1/16BC^2-2xx1/2BCxx1/2BC`
`AD^2=AC^2+1/16BC^2-BC^2`
We know that AB = BC = AC
`AD^2=BC^2+1/16BC^2-BC^2`
`AD^2=(16BC^2+1BC^2-4BC^2)/16`
`AD^2=13/16BC^2`
We know that `DC = 1/4 BC`
`4DC=BC`
Substitute `4DC=BC` in `AD^2 =13/16BC^2 ` we get
`AD^2 = 13/16xx(4DC)^2`
`AD^2 = 13/16xx16DC^2`
`AD^2 = 13/16xx16xxDC^2`
`AD^2=13DC^2`
Hence we have proved that `AD^2 = 13 DC^2`
APPEARS IN
RELATED QUESTIONS
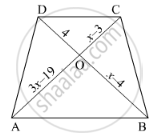
In below figure, AB || CD. If OA = 3x – 19, OB = x – 4, OC = x – 3 and OD = 4, find x.
In each of the following figures, you find who triangles. Indicate whether the triangles are similar. Give reasons in support of your answer.

In the given figure,

AB || DC prove that
DM × BV = BM ✕ DU
In ∆ABC, AD is a median. Prove that AB2 + AC2 = 2AD2 + 2DC2.
State SAS similarity criterion.
If ABC and DEF are similar triangles such that ∠A = 47° and ∠E = 83°, then ∠C =
In the given figure the measure of ∠D and ∠F are respectively

In ∆ABC, a line XY parallel to BC cuts AB at X and AC at Y. If BY bisects ∠XYC, then
∆ABC is a right triangle right-angled at A and AD ⊥ BC. Then, \[\frac{BD}{DC} =\]
In an isosceles triangle ABC if AC = BC and AB2 = 2AC2, then ∠C =
