Advertisements
Advertisements
Question
A wooden toy is in the shape of a cone mounted on a cylinder, as shown in the figure. The total height of the toy is 26 cm, while the height of the conical part is 6 cm. The diameter of the base of the conical part is 5 cm and that of the cylindrical part is 4 cm. The conical part and the cylindrical part are respectively painted red and white. Find the area to be painted by each of these colours. `["Take" pi = 22/7]`

Solution
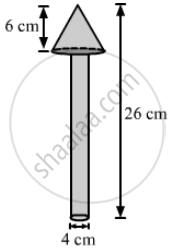
we have,
the base radius of the conical part, r = 5/2= 2.5 cm,
the base radius of the cylindrical part, R = 4/2 = 2 cm
the total height of the toy = 26 cm,
the height of the conical part, h = 6 cm
Also, the height of the cylindrical part , H=26-6 = 20 cm
And, the slant height of the conical part, `l = sqrt(r^2+ h^2) = sqrt (2.5^2+6^2) = sqrt(6.25+36)=sqrt(42.25) = 6.5 cm `
Now,
The area to be painted by red colour = CSA of cone + Area of base of conical part -Area base of cylinderical part
`= pirl+pir^2-piR^2`
`= 22/7xx2.5xx6.5+22/7xx2.5xx2.5-22/7xx2xx2`
`=22/7xx 16.25 +22/7xx6.25- 22/7xx4`
`= 22/7xx(16.25+6.25-4)`
`=22/7xx18.5`
≈ 58.14 cm2
Also,
The area to be painted by white colour = CSA of cylinder + Area of base of cylinder
`= 2piRH + piR^2`
`=piR(2H+R)`
`=22/7xx2xx(2xx20+2)`
`=22/7xx2xx42`
= 264 cm2
APPEARS IN
RELATED QUESTIONS
Water in a canal, 6 m wide and 1.5 m deep, is flowing at a speed of 4 km/h. How much area will it irrigate in 10 minutes, if 8 cm of standing water is needed for irrigation?
In Fig. 4, from the top of a solid cone of height 12 cm and base radius 6 cm, a cone of height 4 cm is removed by a plane parallel to the base. Find the total surface area of the remaining solid. (Use `pi=22/7` and `sqrt5=2.236`)

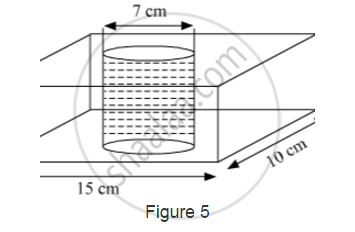
In Fig. 5, from a cuboidal solid metallic block, of dimensions 15cm ✕ 10cm ✕ 5cm, a cylindrical hole of diameter 7 cm is drilled out. Find the surface area of the remaining block [Use
`pi=22/7`]
A toy is in the shape of a right circular cylinder with a hemisphere on one end and a cone on the other. The radius and height of the cylindrical part are 5 cm and 13 cm respectively.The radii of the hemispherical and conical parts are the same as that of the cylindrical part.Find the surface area of the toy if the total height of the toy is 30 cm.
A tent consists of a frustum of a cone capped by a cone. If the radii of the ends of the frustum be 13 m and 7 m , the height of the frustum be 8 m and the slant height of the conical cap be 12 m, find the canvas required for the tent. (Take : π = 22/7)
A bucket made of aluminum sheet is of height 20cm and its upper and lower ends are of radius 25cm an 10cm, find cost of making bucket if the aluminum sheet costs Rs 70 per
100 cm2
A vessel is in the form of hemispherical bowl surmounted by a hollow cylinder of same diameter. The diameter of the hemispherical bowl is 14 cm and the total height of the vessel is 13 cm. Find the total surface area of the vessel. `[\text{Use}pi=22/7]`
A solid sphere of radius r is melted and cast into the shape of a solid cone of height r, the radius of the base of the cone is
In a right circular cone, the cross-section made by a plane parallel to the base is a
The shape of a gilli, in the gilli-danda game (see figure), is a combination of ______.
