Advertisements
Advertisements
Question
AB and CD are the smallest and largest sides of a quadrilateral ABCD. Out of ∠B and ∠D decide which is greater.
Solution
Given: In quadrilateral ABCD, AB is the smallest and CD is the largest side
To find: ∠B > ∠D or ∠D > ∠B.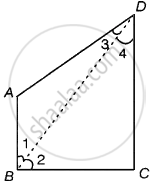
Construction: Join BD.
Now, in ΔABD, AD > AB ...[Since, AB is the smallest side in ABCD]
⇒ ∠1 > ∠3 [Angle opposite to larger side is greater] ...(i)
In ΔBCD, CD > BC ...[Since, CD is the largest side in ABCD]
⇒ ∠2 > ∠4 [Angle opposite to larger side is greater] ...(ii)
On adding equations (i) and (ii), we get
∠1 + ∠2 > ∠3 + ∠4
Hence, ∠B > ∠D
APPEARS IN
RELATED QUESTIONS
In a ΔABC, if ∠A = 55°, ∠B = 40°, find ∠C.
Is the following statement true and false :
A triangle can have two obtuse angles.
Is the following statement true and false :
An exterior angle of a triangle is equal to the sum of the two interior opposite angles.
In the given figure, AB divides ∠DAC in the ratio 1 : 3 and AB = DB. Determine the value of x.
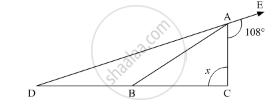
An exterior angle of a triangle is 108° and its interior opposite angles are in the ratio 4 : 5. The angles of the triangle are
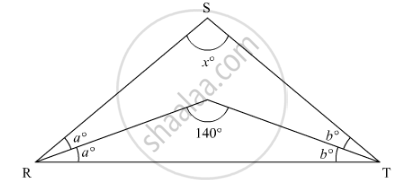
In ΔRST (See figure), what is the value of x?
One angle of a triangle is 61° and the other two angles are in the ratio `1 1/2: 1 1/3`. Find these angles.
The angle of a vertex of an isosceles triangle is 100°. Find its base angles.
The length of the three segments is given for constructing a triangle. Say whether a triangle with these sides can be drawn. Give the reason for your answer.
15 cm, 20 cm, 25 cm
Bisectors of the angles B and C of an isosceles triangle with AB = AC intersect each other at O. BO is produced to a point M. Prove that ∠MOC = ∠ABC.
