Advertisements
Advertisements
Question
Draw a triangle ABC in which BC = 6 cm, CA = 5 cm and AB = 4 cm. Construct a triangle similar to it and of scale factor `5/3`.
Solution
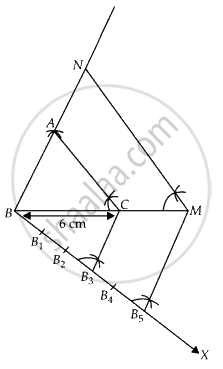
Steps of construction:
- Draw a line segment BC = 6 cm.
- Taking B and C as centres, draw two arcs of radii 4 cm and 5 cm intersecting each other at A.
- Join BA and CA. ∆ABC is the required triangle.
- From B, draw any ray BX downwards making at acute angle.
- Mark five points B1, B2, B3, B4 and B5 on BX, such that BB1 = B1B2 = B2B3 = B3B4 = B4B5.
- Join B3C and from B5 draw B5M || B3C intersecting the extended line segment BC at M.
- From point M draw MN || CA intersecting the extended line segment BA at N.
Then, ∆NBM is the required triangle whose sides is equal to `5/3` of the corresponding sides of the ∆ABC.
Hence, ∆NBM is the required triangle.
APPEARS IN
RELATED QUESTIONS
Write down the equation of a line whose slope is 3/2 and which passes through point P, where P divides the line segment AB joining A(-2, 6) and B(3, -4) in the ratio 2 : 3.
Construct the circumcircle and incircle of an equilateral triangle ABC with side 6 cm and centre O. Find the ratio of radii of circumcircle and incircle.
Construct a Δ ABC in which AB = 6 cm, ∠A = 30° and ∠B = 60°, Construct another ΔAB’C’ similar to ΔABC with base AB’ = 8 cm.
Draw a right triangle in which sides (other than the hypotenuse) are of lengths 8 cm and 6 cm. Then construct another triangle whose sides are 3/4 times the corresponding sides of the first triangle.
Find the ratio in which point P(k, 7) divides the segment joining A(8, 9) and B(1, 2). Also find k.
Find the ratio in which the segment joining the points (1, –3) and (4, 5) is divided by the x-axis? Also, find the coordinates of this point on the x-axis.
Choose the correct alternative:
∆ABC ∼ ∆AQR. `"AB"/"AQ" = 7/5`, then which of the following option is true?
To divide a line segment AB in the ratio p : q (p, q are positive integers), draw a ray AX so that ∠BAX is an acute angle and then mark points on ray AX at equal distances such that the minimum number of these points is ______.
Construction of similar polygons is similar to that of construction of similar triangles. If you are asked to construct a parallelogram similar to a given parallelogram with a given scale factor, which of the given steps will help you construct a similar parallelogram?
Draw a triangle ABC in which AB = 4 cm, BC = 6 cm and AC = 9 cm. Construct a triangle similar to ∆ABC with scale factor `3/2`. Justify the construction. Are the two triangles congruent? Note that all the three angles and two sides of the two triangles are equal.
