Advertisements
Advertisements
Question
Find the magnitude of the electric field at a point 4 cm away from a line charge of density 2 × 10-6 Cm-1.
Solution
Given:
Charge density of the line containing charge, λ = 2 × 10-6 C/m-1
We need to find the electric field at a distance of 4 cm away from the line charge.
We take a Gaussian surface around the line charge of cylindrical shape of radius r = 4 ×10-2 m and height l.
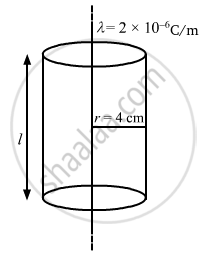
The charge enclosed by the Gaussian surface, qen = λl
Let the magnitude of the electric field at a distance of 4 cm away from the line charge be E.
Thus, net flux through the Gaussian surface, `phi = oint vec"E" . vec"d.s"`
There will be no flux through the circular bases of the cylinder; there will be flux only from the curved surface.
The electric field lines are directed radially outward, from the line charge to the cylinder.
Therefore, the lines will be perpendicular to the curved surface.
Thus,
`phi = oint vec"E" . vec "d.s" = "E" oint "ds" `
`=> phi = "E" xx 2 pi "r""l"`
Applying Gauss's theorem,
`phi = (q_"en")/∈_0`
`"E" xx 2 pi "r""l" =( λ"l")/∈_0`
`"E" = λ/(2 pi "r" ∈_0) `
`"E" = (2xx10^-6)/ (2 xx 3.14 xx8.85 xx 10 ^-12 xx 4 xx 10^-2)`
E = 8.99 × 105 N/C
APPEARS IN
RELATED QUESTIONS
plot a graph showing the variation of current density (j) versus the electric field (E) for two conductors of different materials. What information from this plot regarding the properties of the conducting material, can be obtained which can be used to select suitable materials for use in making (i) standard resistance and (ii) connecting wires in electric circuits?
Two identical circular loops 1 and 2 of radius R each have linear charge densities −λ and +λ C/m respectively. The loops are placed coaxially with their centres `Rsqrt3` distance apart. Find the magnitude and direction of the net electric field at the centre of loop 1.
A metallic particle with no net charge is placed near a finite metal plate carrying a positive charge. The electric force on the particle will be
A charge Q is uniformly distributed over a rod of length l. Consider a hypothetical cube of edge l with the centre of the cube at one end of the rod. Find the minimum possible flux of the electric field through the entire surface of the cube.
Show that there can be no net charge in a region in which the electric field is uniform at all points.
The electric field in a region is given by `vec"E" = ("E"_0 "x")/"l" vec"i".`
Find the charge contained inside the cubical volume bound by the surfaces
x =0, x =a, y=0, y=a, z=0 and z=a. Take
`"E"_0 = 5 xx 10^3 "N""C"^-1 , "l" =2 "cm" " and" " a" = 1 "cm" `
Consider the following very rough model of a beryllium atom. The nucleus has four protons and four neutrons confined to a small volume of radius 10−15 m. The two 1 selectrons make a spherical charge cloud at an average distance of 1⋅3 ×10−11 m from the nucleus, whereas the two 2 s electrons make another spherical cloud at an average distance of 5⋅2 × 10−11 m from the nucleus. Find three electric fields at (a) a point just inside the 1 s cloud and (b) a point just inside the 2 s cloud.
A charged particle with a charge of −2⋅0 × 10−6 C is placed close to a non-conducting plate with a surface charge density of 4.0 × 10-6Cm0-2. Find the force of attraction between the particle and the plate.
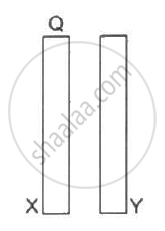
Two conducting plates X and Y, each with a large surface area A (on one side), are placed parallel to each other, as shown in the following figure . Plate X is given a charge Q,whereas the other is kept neutral. Find (a) the surface charge density at the inner surface of plate X (b) the electric field at a point to the left of the plates (c) the electric field at a point in between the plates and (d) the electric field at a point to the right of the plates.
A uniform electric field of 10 N C−1 exists in the vertically downward direction. Find the increase in the electric potential as one goes up through a height of 50 cm.
Some equipotential surface is shown in the figure. What can you say about the magnitude and the direction of the electric field?
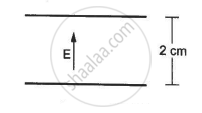
An electric field of magnitude 1000 NC−1 is produced between two parallel plates with a separation of 2.0 cm, as shown in the figure. (a) What is the potential difference between the plates? (b) With what minimum speed should an electron be projected from the lower place in the direction of the field, so that it may reach the upper plate? (c) Suppose the electron is projected from the lower place with the speed calculated in part (b). The direction of projection makes an angle of 60° with the field. Find the maximum height reached by the electron.
A uniform field of 2.0 NC−1 exists in space in the x-direction. (a) Taking the potential at the origin to be zero, write an expression for the potential at a general point (x, y, z). (b) At which point, the potential is 25 V? (c) If the potential at the origin is taken to be 100 V, what will be the expression for the potential at a general point? (d) What will be the potential at the origin if the potential at infinity is taken to be zero? Is it practical to choose the potential at infinity to be zero?
A simple pendulum consists of a small sphere of mass m suspended by a thread of length l. The sphere carries a positive charge q. The pendulum is placed in a uniform electric field of strength E directed vertically downwards. Find the period of oscillation of the pendulum due to the electrostatic force acting on the sphere, neglecting the effect of the gravitational force.
When a comb rubbed with dry hair attracts pieces of paper. This is because the ______.
A charge Q is applied to a conducting sphere of radius R. At the sphere's centre, the electric potential and electric field are respectively
Pick out the statement which is incorrect
Two charged conducting spheres of radii a and b are connected to each other by a wire. Find the ratio of the electric fields at their surfaces.
