Advertisements
Advertisements
Question
Find the value of 'A', if 2cos 3A = 1
Solution
2cos 3A = 1
⇒ cos 3A = `(1)/(2)`
⇒ cos 3A = cos60°
⇒ 3A = 60°
⇒ A = 20°.
APPEARS IN
RELATED QUESTIONS
State for any acute angle θ whether sin θ increases or decreases as θ increases
If sin 3A = 1 and 0 < A < 90°, find sin A
If θ = 30°, verify that: sin2θ = `(2tanθ)/(1 ++ tan^2θ)`
If θ = 15°, find the value of: cos3θ - sin6θ + 3sin(5θ + 15°) - 2 tan23θ
If A = B = 60°, verify that: sin(A - B) = sinA cosB - cosA sinB
If A = B = 60°, verify that: tan(A - B) = `(tan"A" - tan"B")/(1 + tan"A" tan"B"")`
Find the value of 'x' in each of the following:
Find the length of AD. Given: ∠ABC = 60°, ∠DBC = 45° and BC = 24 cm.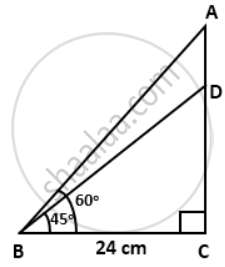
In the given figure, AB and EC are parallel to each other. Sides AD and BC are 1.5 cm each and are perpendicular to AB. Given that ∠AED = 45° and ∠ACD = 30°. Find:
a. AB
b. AC
c. AE
If A + B = 90°, prove that `(tan"A" tan"B" + tan"A" cot"B")/(sin"A" sec"B") - (sin^2"B")/(cos^2"A")` = tan2A
