Advertisements
Advertisements
Question
Find the value of 'x' in each of the following:
Solution
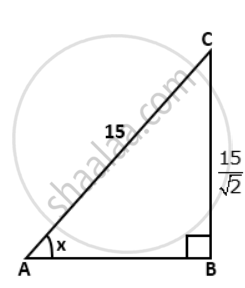
From the figure, we have
sin x = `"BC"/"AC"`
⇒ sin x = `(15/sqrt(2))/(15)`
⇒ sin x = `(1)/sqrt(2)`
⇒ sin x = sin45°
⇒ x = 45°.
APPEARS IN
RELATED QUESTIONS
If 4 sin2 θ - 1= 0 and angle θ is less than 90°, find the value of θ and hence the value of cos2 θ + tan2θ.
If sin 3A = 1 and 0 < A < 90°, find sin A
From the given figure,
find:
(i) cos x°
(ii) x°
(iii) `(1)/(tan^2 xx°) – (1)/(sin^2xx°)`
(iv) Use tan xo, to find the value of y.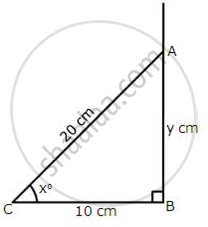
Solve for x : 2 cos 3x - 1 = 0
Solve the following equations for A, if `sqrt3` tan A = 1
Find the magnitude of angle A, if 2 cos2 A - 3 cos A + 1 = 0
Solve for x : cos `(x/(2)+10°) = (sqrt3)/(2)`
If tan4θ = cot(θ + 20°), find the value of θ if 4θ is an acute angle.
If A, B and C are interior angles of ΔABC, prove that sin`(("A" + "B")/2) = cos "C"/(2)`
Prove the following: sin58° sec32° + cos58° cosec32° = 2
