Advertisements
Advertisements
Question
If A + B = 90°, prove that `(tan"A" tan"B" + tan"A" cot"B")/(sin"A" sec"B") - (sin^2"B")/(cos^2"A")` = tan2A
Solution
A + B = 90°
⇒ B = 90° - A
L.H.S.
= `(tan"A" tan"B" + tan"A" cot"B")/(sin"A" sec"B") - (sin^2"B")/(cos^2"A")`
= `(tan"A" tan(90° - "A") + tan"A" cot(90° - "A"))/(sin"A" "cosec""A") - (sin^2 (90° - "A"))/(cos^2 "A")`
= `(tan"A" cot"A" + tan"A" tan"A")/(sin"A" "cosec""A") - (cos^2"A")/(cos^2"A")`
= `( 1 + tan^2 "A")/(1) - 1`
= 1 + tan2A - 1
= tan2A
= R.H.S.
APPEARS IN
RELATED QUESTIONS
From the given figure,
find:
(i) cos x°
(ii) x°
(iii) `(1)/(tan^2 xx°) – (1)/(sin^2xx°)`
(iv) Use tan xo, to find the value of y.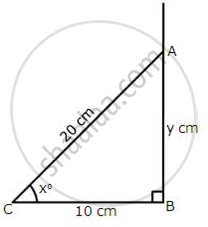
If 3 tan A - 5 cos B = `sqrt3` and B = 90°, find the value of A
Solve for x : cos (2x - 30°) = 0
Evaluate the following: `((sin3θ - 2sin4θ))/((cos3θ - 2cos4θ))` when 2θ = 30°
If A = B = 60°, verify that: sin(A - B) = sinA cosB - cosA sinB
In a trapezium ABCD, as shown, AB ‖ DC, AD = DC = BC = 24 cm and ∠A = 30°. Find: length of AB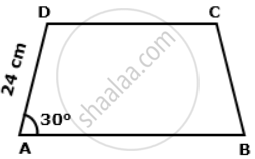
Find:
a. BC
b. AD
c. AC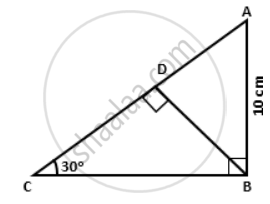
Find the value 'x', if: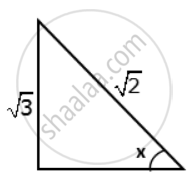
Prove the following: tanθ tan(90° - θ) = cotθ cot(90° - θ)
Prove the following: sin230° + cos230° = `(1)/(2)sec60°`
