Advertisements
Advertisements
Question
For what value of k will the point (3, −k) lie on the line 9x + 4y = 3?
Solution
The given equation of the line is 9x + 4y = 3.
Put x = 3 and y = −k, we have:
9(3) + 4(−k) = 3
27 − 4k = 3
4k = 27 − 3 = 24
k = `24/4`
k = 6
APPEARS IN
RELATED QUESTIONS
A(-1, 3), B(4, 2) and C(3, -2) are the vertices of a triangle.
1) Find the coordinates of the centroid G of the triangle
2) Find the equation of the line through G and parallel to AC
Find, which of the following points lie on the line x – 2y + 5 = 0 :
(1, 3)
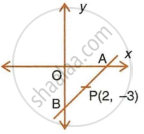
A and B are two points on the x-axis and y-axis respectively. P(2, −3) is the mid point of AB. Find the
- co-ordinates of A and B
- slope of line AB
- equation of line AB.
The line 2x - 5y + 31 = 0 bisects the join of (-4,5) and (P, 9). Find the value of p.
Find the equation of a line passing through (3,7) and making an angle of 60° with the negative direction of the x-axis.
Find the equation of a line which is inclined to x axis at an angle of 60° and its y – intercept = 2.
Find the equation of a line with slope 1 and cutting off an intercept of 5 units on Y-axis.
Find the equation of a straight line which cuts an intercept of 5 units on Y-axis and is parallel to the line joining the points (3, – 2) and (1, 4).
Find the equation of the line passing through (0, 4) and parallel to the line 3x + 5y + 15 = 0.
A and B are two points on the x-axis and y-axis respectively.

- Write down the coordinates of A and B.
- P is a point on AB such that AP : PB = 1 : 1.
Using section formula find the coordinates of point P. - Find the equation of a line passing through P and perpendicular to AB.
