Advertisements
Advertisements
Question
I have three sides. One of my angle measures 15°. Another has a measure of 60°. What kind of a polygon am I? If I am a triangle, then what kind of triangle am I?
Solution
A polygon having three sides is a triangle.
Given that the two angles are of measure 15° and 60°
Let the third angle of triangle be x.
∴ 15° + 60° + x = 180° ......[Angle sum property of a triangle]
⇒ x = 180° – 60° – 15°
⇒ x = 105°
Thus, one angle of triangle is an obtuse angle. So, the triangle is an obtuse-angled triangle.
APPEARS IN
RELATED QUESTIONS
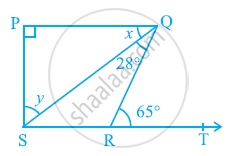
In the given figure, if PQ ⊥ PS, PQ || SR, ∠SQR = 28º and ∠QRT = 65º, then find the values of x and y.
In the given figure, the side QR of ΔPQR is produced to a point S. If the bisectors of ∠PQR and ∠PRS meet at point T, then prove that ∠QTR = 1/2∠QPR.

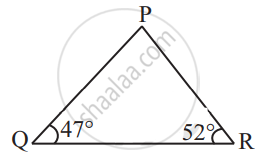
In the given figure find m∠P.
If the three angles of a triangle are in the ratio 3 : 5 : 4, then find them
Prove that a triangle must have atleast two acute angles.
If two angles of a triangle are 60° each, then the triangle is ______.
In ∆ABC, ∠Α = 100°, AD bisects ∠A and AD ⊥ BC. Then, ∠B is equal to ______.
The sum of the measures of three angles of a triangle is greater than 180°.
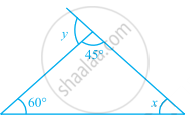
In the given figure, find the measures of ∠x and ∠y.
Each of the two equal angles of an isosceles triangle is four times the third angle. Find the angles of the triangle.
