Advertisements
Advertisements
Question
In the given figure, find the measures of ∠x and ∠y.
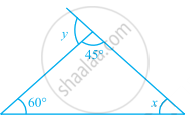
Solution

In ∆ABC,
∠BAC + ∠ABC + ∠ACB = 180° ......[Angle sum property]
⇒ 45° + 60° + x = 180°
⇒ x = 180° – 45° – 60° = 75° ......(i)
Now, ∠BAD = ∠ABC + ∠ACB ......[Exterior angle property]
⇒ y = 60° + x
⇒ y = 60° + 75° = 135° ......[Using (i)]
Thus, x = 75° and y = 135°
APPEARS IN
RELATED QUESTIONS
Find the value of the unknown x in the following diagram:

In the following triangle, find the value of x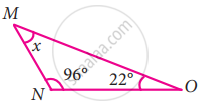
In the following triangle, find the value of x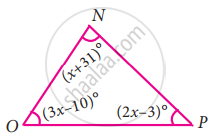
In ∆ABC, if ∠B is 3 times ∠A and ∠C is 2 times ∠A, then find the angle
In ∆DEF, ∠F = 48°, ∠E = 68° and bisector of ∠D meets FE at G. Find ∠FGD
Can a triangle have two obtuse angles? Give reason for your answer.
Bisectors of interior ∠B and exterior ∠ACD of a ∆ABC intersect at the point T. Prove that `∠BTC = 1/2 ∠BAC`.
Triangle DEF of the given figure is a right triangle with ∠E = 90°. What type of angles are ∠D and ∠F?
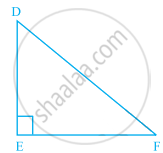
The sum of the measures of three angles of a triangle is greater than 180°.
In the given figure, if ST = SU, then find the values of x and y.

