Advertisements
Advertisements
Question
If the three angles of a triangle are in the ratio 3 : 5 : 4, then find them
Solution
Given three angles of the triangle are in the ratio 3 : 5 : 4.
Let the three angle be 3x, 5x and 4x.
By angle sum property of a triangle, we have
3x + 5x + 4x = 180°
12x = 180°
x = `(180^circ)/12`
x = 15°
∴ The angle are 3x = 3 × 15° = 45°
5x = 5 × 15° = 75°
4x = 4 × 15° = 60°
Three angles of the triangle are 45°, 75°, 60°
APPEARS IN
RELATED QUESTIONS
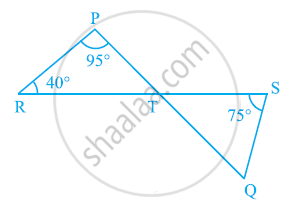
In the given figure, if lines PQ and RS intersect at point T, such that ∠PRT = 40º, ∠RPT = 95º and ∠TSQ = 75º, find ∠SQT.
In ∆RST, ∠S is 10° greater than ∠R and ∠T is 5° less than ∠S, find the three angles of the triangle
A triangle ABC is right angled at A. L is a point on BC such that AL ⊥ BC. Prove that ∠BAL = ∠ACB.
If one angle of a triangle is equal to the sum of the other two angles, the triangle is ______.
In ∆ABC, ∠Α = 100°, AD bisects ∠A and AD ⊥ BC. Then, ∠B is equal to ______.
If one angle of a triangle is equal to the sum of other two, then the measure of that angle is ______.
It is possible to have a triangle in which each angle is greater than 60°.
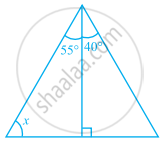
In the given figure, find the value of x.
In a triangle ABC, the measure of angle A is 40° less than the measure of angle B and 50° less than that of angle C. Find the measure of ∠A.
In ΔPQR, if 3∠P = 4∠Q = 6∠R, calculate the angles of the triangle.
