Advertisements
Advertisements
Question
If ABC is an equilateral triangle inscribed in a circle and P be any point on the minor arc BC which does not coincide with B or C, prove that PA is angle bisector of ∠BPC.
Advertisements
Solution
Given: ΔABC is an equilateral triangle inscribed in a circle and P be any point on the minor arc BC which does not coincide with B or C.
To prove: PA is an angle bisector of ∠BPC.
Construction: Join PB and PC.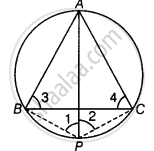
Proof: Since, ΔABC is an equilateral triangle.
∠3 = ∠4 = 60°
Now, ∠1 = ∠4 = 60° ...(i) [Angles in the same segment AB]
∠2 = ∠3 = 60° ...(ii) [Angles in the same segment AC]
∴ ∠1 = ∠2 = 60°
Hence, PA is the bisector of ∠BPC.
Hence proved.
APPEARS IN
RELATED QUESTIONS
A circle touches the side BC of a ΔABC at a point P and touches AB and AC when produced at Q and R respectively. As shown in the figure that AQ = `1/2` (Perimeter of ΔABC).
Fill in the blanks:
Segment of a circle is the region between an arc and __________ of the circle.
Draw different pairs of circles. How many points does each pair have in common? What is the maximum number of common points?
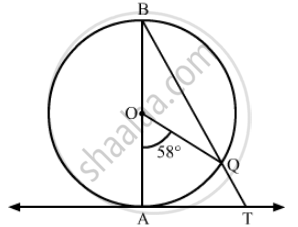
In the given figure, O is the centre of the circle. If ∠AOB = 140° and ∠OAC = 50°; find:
- ∠ACB,
- ∠OBC,
- ∠OAB,
- ∠CBA.
In the following figure, AB is the diameter of a circle with centre O and CD is the chord with length equal to radius OA.
Is AC produced and BD produced meet at point P; show that ∠APB = 60°
In the given figure, PO \[\perp\] QO. The tangents to the circle at P and Q intersect at a point T. Prove that PQ and OTare right bisector of each other.
In Fig. 8.79, PQ is a tangent from an external point P to a circle with centre O and OP cuts the circle at T and QOR is a diameter. If ∠POR = 130° and S is a point on the circle, find ∠1 + ∠2.
Circles with centers A, B and C touch each other externally. If AB = 36, BC = 32, CA = 30, then find the radii of each circle.
In the following figure, if ∠OAB = 40º, then ∠ACB is equal to ______.
Say true or false:
Two diameters of a circle will necessarily intersect.
