Question

In a quadrilateral ABCD, ∠A + ∠D = 90°. Prove that AC² + BD² = AD² + BC² 

[Hint: Produce AB and DC to meet at E.]

Answer 1

Given: Quadrilateral ABCD, in which ∠A + ∠D = 90°

To prove: AC² + BD² = AD² + BC²

Construct: Produce AB and CD to meet at E

Also join AC and BD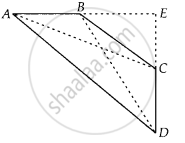

Proof: In ∆AED, ∠A + ∠D = 90°   ...[Given]

∴ ∠E = 180° – (∠A + ∠D) = 90°  ...[∵ Sum of angles of a triangle = 180°]

Then, by Pythagoras theorem,

AD² = AE² + DE²

In ∆BEC, by Pythagoras theorem,

BC² = BE² + EC²

On adding both equations, we get

AD² + BC² = AE² + DE² + BE² + CE²  ...(i)

In ∆AEC, by Pythagoras theorem,

AC² = AE² + CE²

And in ∆BED, by Pythagoras theorem,

BD² = BE² + DE²

On adding both equations, we get

AC² + BD² = AE² + CE² + BE² + DE²  ...(ii)

From equations (i) and (ii),

AC² + BD² = AD² + BC²

Hence proved.