Advertisements
Advertisements
Question
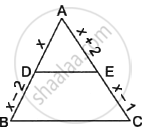
In a ΔABC, D and E are points on the sides AB and AC respectively. For the following case show that DE || BC
AB = 5.6cm, AD = 1.4cm, AC= 7.2 cm and AE = 1.8 cm.
Solution
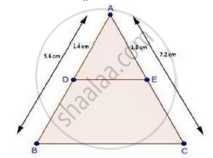
We have,
AB = 5.6 cm, AD = 1.4 cm, AC = 7.2 cm and AE = 1.8 cm
∴ DB = AB – AD
= 5.6 – 1.4
⇒ DB = 4.2 cm
And, EC = AC – AE
= 7.2 – 1.8
⇒ EC = 5.4 cm
Now, `"AD"/"DB"=1.4/4.2=1/3` [∵ DB = 4.2 cm]
And, `"AE"/"EC"=1.8/5.4=1/3` [∵ EC = 5.4 cm]
Thus, DE divides sides AB and AC of ΔABC in the same ratio.
Therefore, by the converse of basic proportionality theorem,
We have, DE || BC
APPEARS IN
RELATED QUESTIONS
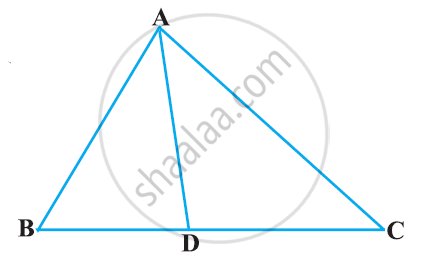
In the given figure, D is a point on side BC of ΔABC such that ∠ADC=∠BAC . Prove that AD is the bisector of ∠BAC.
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If `"AD"/"DB"=2/3` and AC = 18 cm, find AE
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 8x − 7, DB = 5x − 3, AE = 4x − 3 and EC = (3x − 1), find the value of x.
In the adjoining figure, ABC is a triangle in which AB = AC. IF D and E are points on AB and AC respectively such that AD = AE, show that the points B, C, E and D are concyclic.

Find the height of an equilateral triangle of side 12cm.
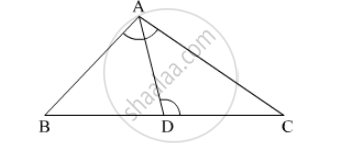
In the given figure, in ∆ABC, point D on side BC is such that, ∠BAC = ∠ADC. Prove that, CA2 = CB × CD
In figure, PA, QB, RC and SD are all perpendiculars to a line l, AB = 6 cm, BC = 9 cm, CD = 12 cm and SP = 36 cm. Find PQ, QR and RS.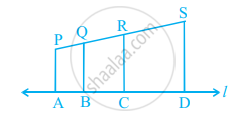
In figure, line segment DF intersect the side AC of a triangle ABC at the point E such that E is the mid-point of CA and ∠AEF = ∠AFE. Prove that `(BD)/(CD) = (BF)/(CE)`.
In the given figure, ABC is a triangle in which DE||BC. If AD = x, DB = x – 2, AE = x + 2 and EC = x – 1, then find the value of x.
State and prove Basic Proportionality theorem.
