Advertisements
Advertisements
Question
In a ΔABC, P and Q are points on sides AB and AC respectively, such that PQ || BC. If AP = 2.4 cm, AQ = 2 cm, QC = 3 cm and BC = 6 cm, find AB and PQ.
Solution

We have || BC
Therefore, by BPT
We have,
`"AP"/"PB"="AQ"/"QC"`
`2.4/"PB"=2/3`
`rArr"PB"=(3xx2.4)/2=(3xx24)/2=(3xx6)/5=18/5`
⇒ PB = 3.6 cm
Now, AB = AP + PB
= 2.4 + 3.6 = 6cm
Now, In ΔAPQ and ΔABC
∠A = ∠A [common]
∠APQ = ∠ABC [∵ PQ || BC ⇒ Corresponding angles are equal]
⇒ ΔAPQ ~ ΔABC [By AA criteria]
`rArr"AB"/"AP"="BC"/"PQ"` [corresponding sides of similar triangles are proportional]
`rArr"PQ"=(6xx2.4)/6`
⇒ PQ = 2.4 cm
Hence, AB = 6 cm and PO = 2.4 cm
APPEARS IN
RELATED QUESTIONS
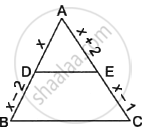
D and E are points on the sides AB and AC respectively of a ΔABC such that DE║BC. Find the value of x, when
AD = x cm, DB = (x – 2) cm, AE = (x + 2) cm and EC = (x – 1) cm.
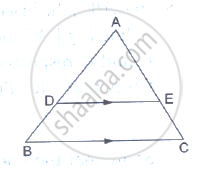
ABCD is a parallelogram in which P is the midpoint of DC and Q is a point on AC such that CQ = `1/4` AC. If PQ produced meets BC at R, prove that R is the midpoint of BC.

A guy wire attached to a vertical pole of height 18 m is 24m long and has a stake attached to the other end. How far from the base of the pole should the stake be driven so that the wire will be taut?
In the given figure, D is the midpoint of side BC and AE⊥BC. If BC = a, AC = b, AB = c, AD = p and AE = h, prove that
(i)`B^2=p^2+ax+a^2/x`
(ii)` c^2=p^2-ax+a^2/x`
(iii) `b^2+c^2=2p^2+a^2/2`
(iv)`b^2-c^2=2ax`

State the basic proportionality theorem.
State the midpoint theorem
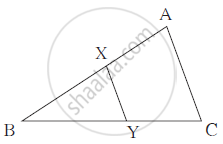
In the adjoining figure,
seg XY || seg AC, If 3AX = 2BX
and XY = 9 then find the length of AC.
From fig., seg PQ || side BC, AP = x + 3, PB = x – 3, AQ = x + 5, QC = x – 2, then complete the activity to find the value of x.
In ΔPQB, PQ || side BC
`"AP"/"PB" = "AQ"/(["______"])` ...[______]
`(x + 3)/(x - 3) = (x + 5)/(["______"])`
(x + 3) [______] = (x + 5)(x – 3)
x2 + x – [______] = x2 + 2x – 15
x = [______]
Find the value of x for which DE || AB in figure.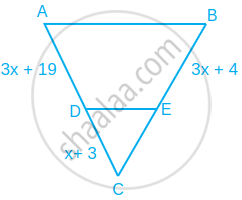
In the given figure, ABC is a triangle in which DE||BC. If AD = x, DB = x – 2, AE = x + 2 and EC = x – 1, then find the value of x.