Advertisements
Advertisements
Question
In ΔABC, seg XY || side AC. If 2AX = 3BX and XY = 9, then find the value of AC.
Solution
Given, 2AX = 3BX
∴ `("AX")/("BX") = 3/2`
`("AX" + "BX")/("BX") = (3 + 2)/2` ......[By componendo]
∴ `("BA")/("BX") = 5/2` [A – X – B] ......(i)
In ΔBCA and ΔBYX,
`{:(∠"BCA" ≅ ∠"BYX"),(∠"BAC" ≅ ∠"BXY"):}}` ......[Corresponding angles]
∴ ΔBCA ∼ ΔBYX ......[By AA test of similarity]
∴ `("BA")/("BX") = ("AC")/("XY")` ......[Corresponding sides of similar triangle]
∴ `5/2 = ("AC")/9`
∴ AC = `(5 xx 9)/2 = 45/2`
∴ AC = 22.5 units
APPEARS IN
RELATED QUESTIONS
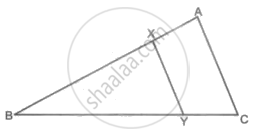
State which pair of triangles in the following figure are similar. Write the similarity criterion used by you for answering the question, and also write the pairs of similar triangles in the symbolic form:
S and T are point on sides PR and QR of ΔPQR such that ∠P = ∠RTS. Show that ΔRPQ ∼ ΔRTS.
In the following figure, altitudes AD and CE of ΔABC intersect each other at the point P. Show that:

ΔABD ∼ ΔCBE
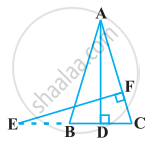
In the following figure, E is a point on side CB produced of an isosceles triangle ABC with AB = AC. If AD ⊥ BC and EF ⊥ AC, prove that ΔABD ∼ ΔECF.
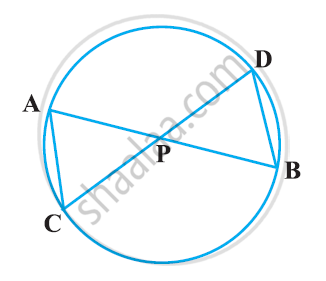
In the given figure, two chords AB and CD intersect each other at the point P. prove that:
(i) ΔAPC ∼ ΔDPB
(ii) AP.BP = CP.DP
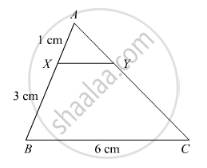
In the following figure, XY || BC. Find the length of XY.
State the AAA-similarity criterion
A ladder 10m long reaches the window of a house 8m above the ground. Find the distance of the foot of the ladder from the base of the wall.
In the given figure, seg AC and seg BD intersect each other in point P and `"AP"/"CP" = "BP"/"DP"`. Prove that, ∆ABP ~ ∆CDP.

In the figure, if DE∥BC, AD = 3cm, BD = 4cm and BC= 14 cm, then DE equals ______.
Two sides and the perimeter of one triangle are respectively three times the corresponding sides and the perimeter of the other triangle. Are the two triangles similar? Why?
Areas of two similar triangles are 36 cm2 and 100 cm2. If the length of a side of the larger triangle is 20 cm, find the length of the corresponding side of the smaller triangle.
A street light bulb is fixed on a pole 6 m above the level of the street. If a woman of height 1.5 m casts a shadow of 3 m, find how far she is away from the base of the pole.
In the given figure, S is a point on side QR of ΔPQR such that ∠QPR = ∠PSR. Use this information to prove that PR2 = QR × SR.
In ΔABC, AP ⊥ BC, BQ ⊥ AC. If AP = 7, BQ = 8 and BC = 12, then find AC.

In the given figure, DE ∥ BC, AE = a units, EC = b units, DE = x units and BC = y units. Which of the following is true?
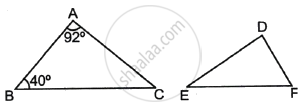
If ΔABC ~ ΔDEF and ∠A = 47°, ∠E = 83°, then ∠C is equal ______.
If ΔABC ∼ ΔDEF such that ∠A = 92° and ∠B = 40°, then ∠F = ?